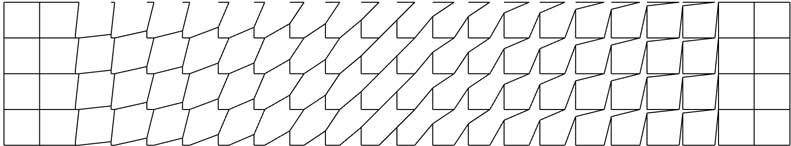
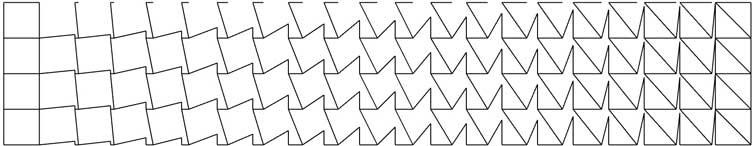
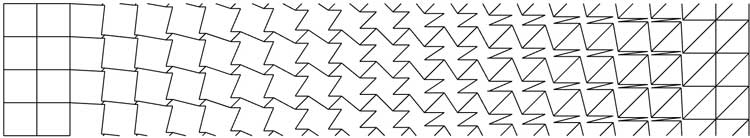
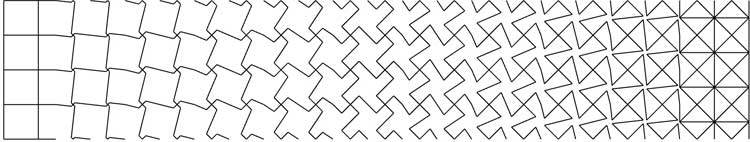
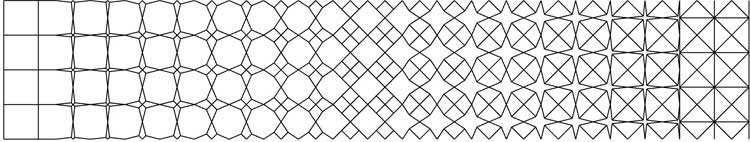
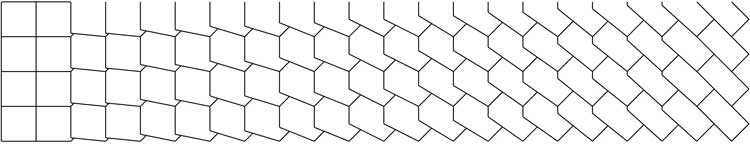
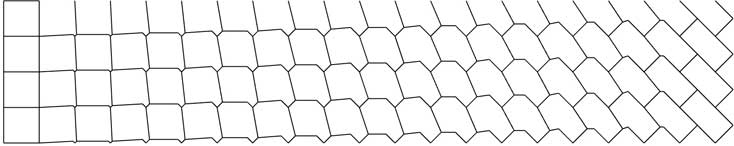
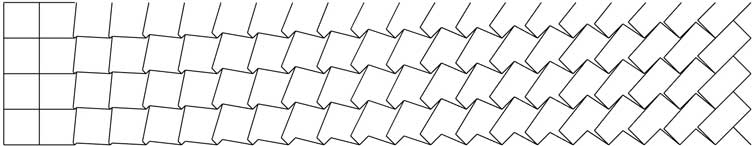
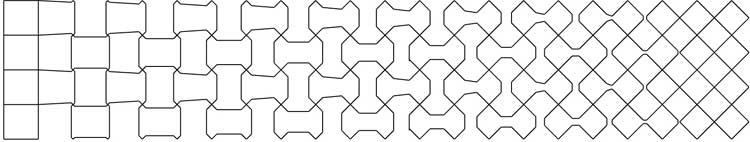
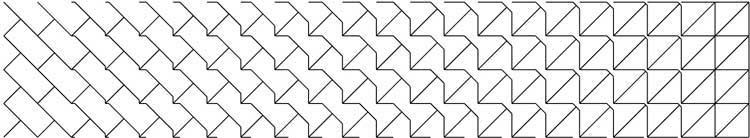
Specific Transitions

I’ll begin by stating that this page, as of this May 2021 writing, like most of the sub parquet deformation pages, is in a state of flux, and so is subject to review and change. Ideally, I would wait and compose a more polished piece, but this may otherwise take some time, perhaps even many years before this would appear. The reason for this is that I am in the midst of extensive parquet deformation studies, of various types (of a periodic random return, but this time caused by external factors, of an article for a forthcoming book, but this went unrealised). Further, I am also in the process of redrawing existing hand-drawn parquet deformations in Rhino, as well as exploring new possibilities offered by computer-drawn diagrams. Essentially, redrawing and study go hand in hand, with improvements in one leading to advances in another. The scope of parquet deformations is wide indeed, much more so that is generally realised, and as is my wont, I investigate the many aspects at whim. Therefore, although ideally I would investigate any one aspect to exhaustion, this would in practice possibly take many years, and even then with no certainty of completion. This being so, and given that in any case I thrive on novelty (at the expense of completion in the normal sense), I have only made an initial study of each aspect. Therefore, this page is typical. That said, I have indeed tried to be as thorough and exact as possible in the circumstances.

Of interest is in what I term as specific transitions. That is, a transition of one tiling polygon to another, such as squares to rectangles. Amazingly, such simple, core value transitions, and others are essentially ignored as a parquet deformation/morph problem. Indeed, aside from myself, the only other person to have studied such specifics is Craig Kaplan, who in his paper ‘Metamorphosis in Escher’s Art’ concerned himself with transitions between both Isohedral and the Laves tilings (as separate entities), largely in the same ‘simple’, or easily stated, goal. However, we approach the problem in different ways. My procedure, formed of an ‘open grid’, can (in this context) be described as effectively unplanned, or even ‘hit or miss’, whilst Kaplan’s is more advanced mathematically, of university-level maths with advanced algorithms, and is focused, with a pre-determined choice of tiles in mind. I title mine as ‘hit or miss’, in that the procedure does not set out to produce any specific transition, but rather is only to be found upon completion of the design process. That said, as these are square-based grids, derivatives can readily be imagined, such as rectangles, or two right-angled triangles set within a square, although the premise of non-specific transitions remains. However, although arguably inefficient compared to Kaplan’s procedure, nonetheless it does produce core-value transitions of aesthetic transitions. Note that there is more than one way to transition between two tiles, and which adds extra interest. For instance, the transitioning tiles can be asymmetrical or mirror images, as well as of different symmetry operations of translation and reflection. Again, aesthetics are involved here. Generally, I prefer transitions with symmetry, as against none.

Note that the parquet deformations are not unique in themselves, but rather that they are taken, or more exactly filtered, from my archive page.

Each specific transition has a brief commentary (for now) as to its merits (or otherwise!). This will later be expanded upon.

Each instance can be interpreted in a dual manner, e.g. ‘Square to two right-angled triangles within a square/Two right-angled triangles within a square to square’. Simply stated, the reverse is possible by simply rotating the parquet deformation 180°, and so rather than in effect duplicating existing examples resulting in a very long listing, I thus list this dual ordering. I may indeed later show this for the sake of easier viewing.

Aside from the general compilation, the above collection can be used to select as according to the best in class, for any one instance, to be the subject of a forthcoming page.

1. Square to Square (1)