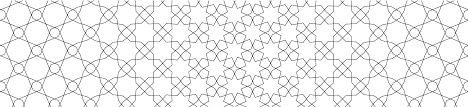
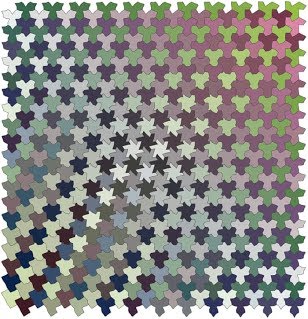
Parquet Deformations of Craig Kaplan

Craig S. Kaplan (1972–), a Canadian computer scientist/mathematician, with an interest in mathematical art and computer graphics, can quite simply be described as the leading light in parquet deformation research. In short, he brings his superior mathematical mind to bear on the topic and shows a whole host of innovative and advanced examples that without the aid of the computer would simply otherwise be impractical. Typically, parquet deformations are portrayed in a ‘simple’ manner, as a linear strip. However, although all well and good (and there is nothing ‘wrong’ with this), other geometric configurations are indeed possible, in which the concept is expanded, and so is thus made arguably more interesting. For instance, as shown by Kaplan below, building upon the one dimension, two dimensions are possible, with a squared format, in which the deformation is ‘continuous allover’; left/right, top/bottom. Another variation they can be turned into a loop, with a circle. And another innovation is that of an Islamic tiling theme, where, due to the somewhat involved nature of the tiling these are shown possible that simply would otherwise be impractical by traditional hand methods. And again, of a similar involved vein in concept, fractals are also shown. The transitions between the Laves tilings are also investigated, as is a study of the isohedral tilings. In addition, there are also complex computer algorithms developed by others applied here (way beyond the typical recreational mathematical knowledge to compile such ‘simple’ linear instances), such as that by Hans Pedersen and Karan Singh. All of which embodies a most impressive body of work. Further, he has written extensively on the subject with a variety of articles, below, all of which all can be considered as must reading (and pleasingly has made these readily accessible).