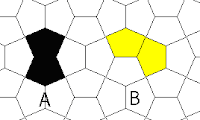
Of interest mathematically is that of the colouration possibilities of the tiles. Here I examine both colouration per se, as an abstract entity (albeit with minimal reference to the in situ tiles for general interest) followed by the in situ pavings, again as a distinct entity. Regarding the coloration as an abstract entity per se, I investigate two aspects. I begin with map-coloured tilings, followed by a variety of contiguous colourings. An open question is to the number of distinct map colorations, using three (being the minimum), four, five, or more colours. As a rule, the more colours the more complex the matter, of which for colourings greater than four are really only of interest academically, these lacking the elegance of the more ‘straightforward’ 3 and (at times) 4-colourings. Of course, a multitude of possibilities are possible when the map colouring restriction is lifted, of which I briefly examine such instances as well, albeit most of these are trivial. Upon having examined colouration per se, I then turn my attention to an examination of the colouring used on the in situ pavings. To the best of my knowledge, surprisingly, colouration per se of this tiling has not been examined, at least to any great extent, as here. I would be indebted to any reader which can refer me to any work in this field, or indeed, may be interested in studying this aspect themselves. Colouration Per Se Map Colourings; 3, 4, 5… Colours
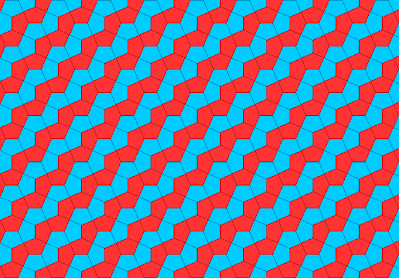
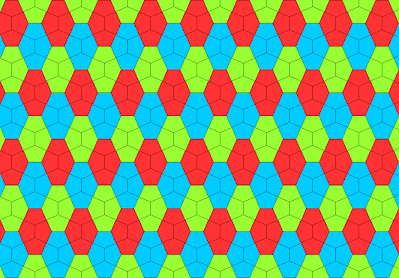
3 Colours The (minimum) 3-colouring is forced, although at first glance this is not obvious, as each colour appears in four orientations, in a not particularly intuitive way. The minimum colouring is easily demonstrated, if not proven here. I'll send on request.
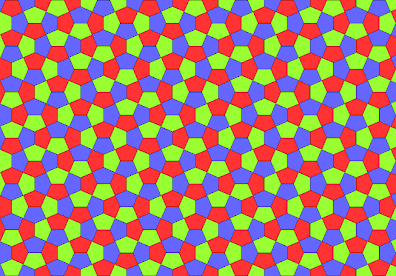 Fig. 1: Three colours, the only possible arrangement. Of interest is that despite this being the minimum colouring, this has yet to be seen in the in situ pavings.
4 Colours
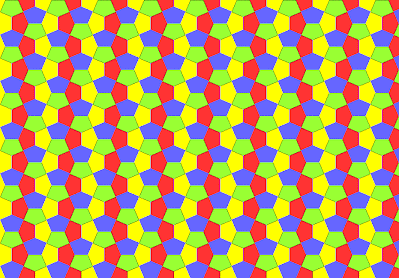
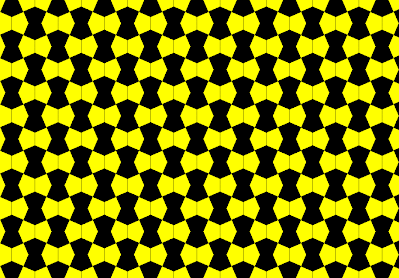
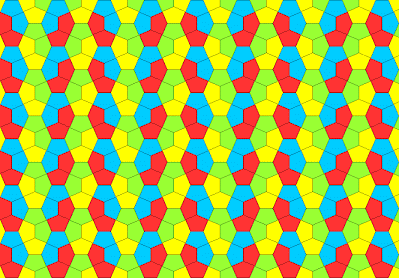
Four colours offer much more variety, of which the colouring scheme is not forced, as with 3-colours above. Unfortunately, I lack the ability to prove the number of distinct colourations, but there appears to be just three; Figs 2a, 2b, 2c, below. By far, Fig. 2a is the easiest to imagine, with one orientation, one colour, of which the colouring scheme is obvious. Figs. 2b, 2c are less intuitive. 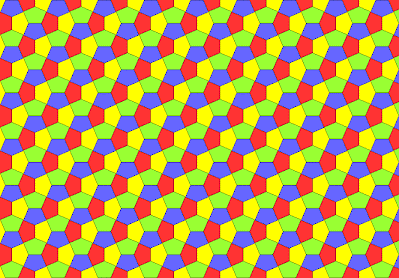 Fig. 2a: All four colours in four orientations of the tile. This is frequently to be seen among the in situ pavings, likely being the most ‘intuitive’ colouring of all, including the 3-colouring. I.e. simply place tiles of the same colour in the same orientation, and the colouration repeats as above, no thought required)
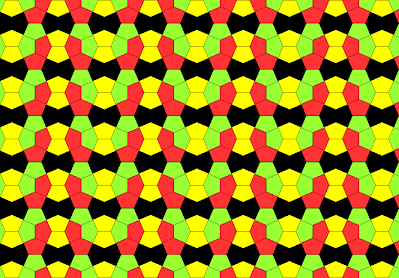
 Fig. 2b: All four colours are all in ‘ribbons’, linked (or joined) at the 90° angle of the pentagon. Not seen in the in situ pavings. Figure 2c: A combination of the above, with blue
and green of the same orientation type (Fig. 2a) and ‘ribbons’ red and yellow (Fig.
2c). Not seen in the in situ pavings.
5 Colours
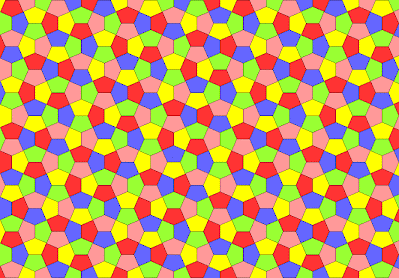
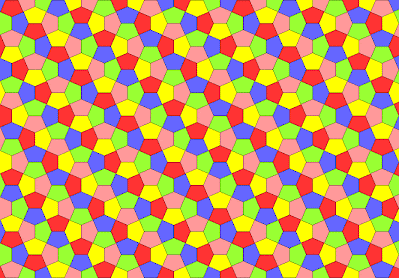
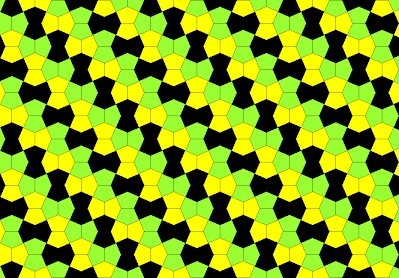
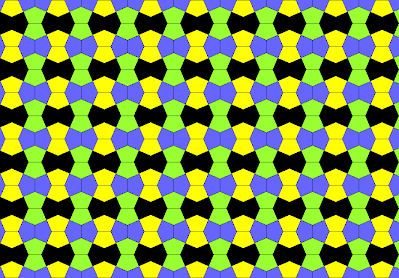
Five colours become a little difficult to analyse. Likely there is more than that shown here, of which these are the work of George Baloglou. Figure 3a: Not seen in the in situ pavings Fig. 3b: All colourings are of diagonals at c. 45°. Not seen in the in situ pavings
Six Colours
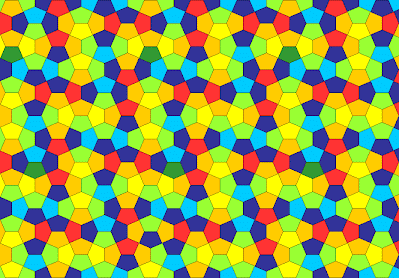
Fig. 4. After George Baloglou. Not seen in the in situ pavings. For a more in-depth analysis of six-colouring possibilities, see George Baloglou's blog:
Contiguous (Fused) Colourings
With the restriction of map colouring relaxed, a whole host of colourations of contiguous colours, or what I term as ‘fused’ below, are possible. Indeed, there are so many possibilities here that they soon become trivial, so much so that I largely gloss over any in-depth analysis here. Undoubtedly, these are weaker than the map-colouring condition, in that the individual pentagons are not so readily seen. Consequently, I show just a few of the possibilities, with occasional comment. Reference is made to two different types of fused pentagon, with (a) ‘Macmillan’ and (b) ‘90°’; this serves for ease of description. ‘Macmillan’ is of the pentagons placed ‘back to back’ (as first described by Macmillan of the in situ pavings, of 1979), whilst ‘90°’ describes the ‘other’ meeting possible, at the 90° angle. To clarify, I show below:
Fig. 5: The two fused instances, ‘Macmillan’ (A) and 90° (B)
(a) Macmillan Types
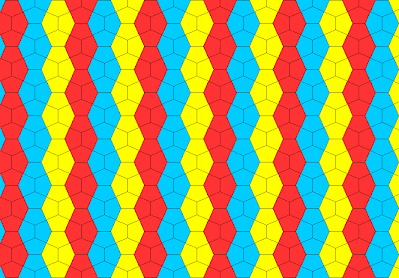
Fig. 6: Macmillan type, two colours. This is undoubtedly the most intuitive of the fusion possibilities. This is frequently to be seen among the in situ pavings (a notable sighting being at the American University in Cairo, and as alluded to above, the most ‘intuitive’ fused instance of all. Fig. 8: Macmillan type, four colours. Not seen in the in situ pavings
(b) 90° Types
Fig. 9: Diagonal. As seen in the in situ pavings, at Heliopolis, outside Caesar’s Place hotel, as reported by Robin Wilson
Fig. 10: 90°, with four colours used. Of the fused type, this is perhaps better than most, it retaining ‘integrity’. Not seen in the in situ pavings
Fig. 11: Macmillan and 90° fusion of two pentagons. Not seen in the in situ pavings
(d) Four Pentagons in a Hexagonal Block
These lack any real interest. Essentially, they can be said to lack imagination
Fig. 12: Columns, 2 colours. Not seen in the in situ pavings
Fig. 13: Columns, 3 colours. Not seen in the in
situ pavings Part 2
In Situ Colourings
Upon having studied in an abstract sense, I now examine the in situ pavings, with the above analysis in mind, and see how these are arranged.
Map Colourings
4 Colours Fig. 15 Typical 4-colouring of Fig 2a This is by far the most frequently seen colouration, likely due to its sheer ease of placement, in that to achieve this all one needs to consider is a ‘same orientation, same colour’ rule. However, again, one would have to ask if the layman would be aware of this. If there was a picture provided, then this could explain its relative frequency. Possibly not, which would explain instances of contiguous colour, likely out of either lack of interest or lack of success with trying to repeat in a regular way, leading to frustration, and so placing the tiles ‘any which way’.
5 Colours A single instance has been found, but the properties of the colouring remains uncertain, due to a small patch, from which one cannot tell if it is regular or not. Upon examination, with isolating each colour to see if there is regularity, it would appear not. However, for reasons as above, this is a provisional statement, subject to change.  Fig. 16 As few tiles are shown, certainly not enough to detemine if the pattern continues, whether this is a regular colouring is uncertain. Upon preliminary investigation, it would appear not. If a regular five colouring was indeed discerned, this would be significant, as it would suggest a mathematician is behind this; beyond all reasonable doubt, a lay person would not go to the time and trouble for such an involved colouring.
Random Colourings
Some instances defy analysis, as upon an apparent attempt at a innovative colouring scheme, the instigator loses his way, and the colouring becomes random.  Fig. 17: Maadi Contiguous Colourings 2 Colours Fig. 19: As defined by Macmillan, in black and white. AUC campus here outside the administration building Fig. 21: As defined by Macmillan, but burgundy and dunn, at the Old Cataract hotel Random Arbitrary Contiguous Colourings 
Fig. 22
Likely the designer was attempting a more 'involved' Macmillan colouring, of three colours, but lost his way
Occasionally seen are colourings where no pretence is made as to regularity, with colours appearing ‘any which way’, but with contiguity. Although such a sight may grate to those who prefer order, one should not forget that most people would have no interest in the subject per se, with the intention of simply paving a given space in the least amount of time and effort. Indeed, the very nature of the tiling, with many variations as to colour would lead this way, with the user giving up in frustration. Such examples may therefore lead to the supposition that no guidance was given to colour arrangement at the time of purchase, but as with much of the study, one cannot be too certain of this hypothesis. Even within this, some attempts at order are made. Here is a multi-coloured butterfly configuration.
Created: 8 February 2013. Last update 21 October 2013 |
Cairo Tiling >