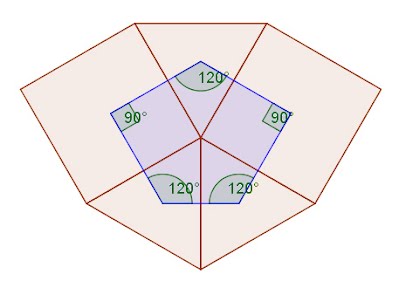
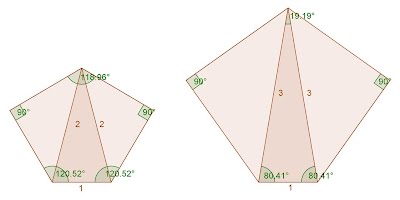
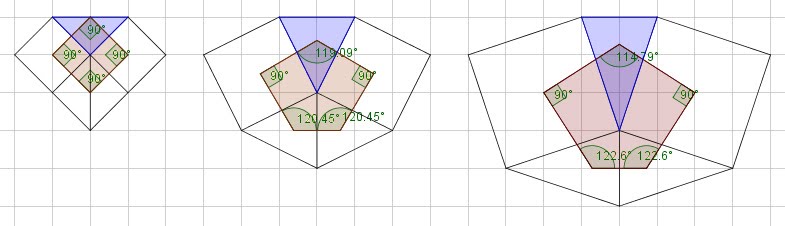
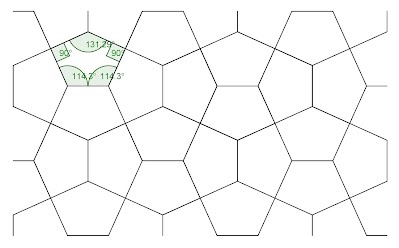
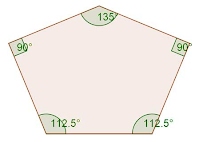
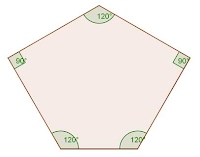
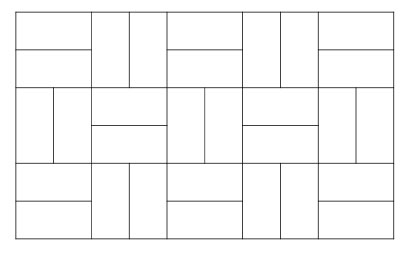
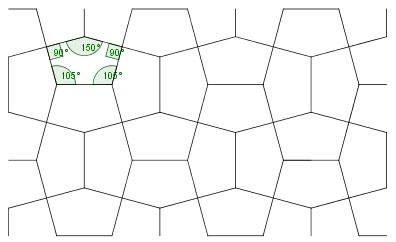
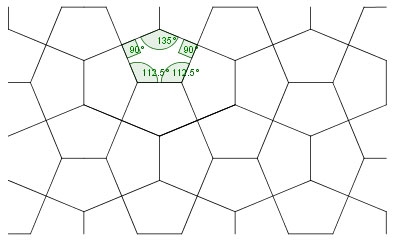
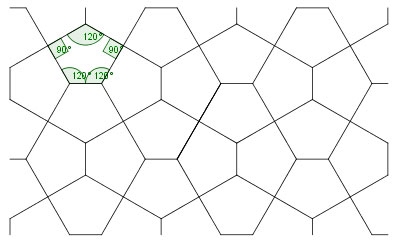
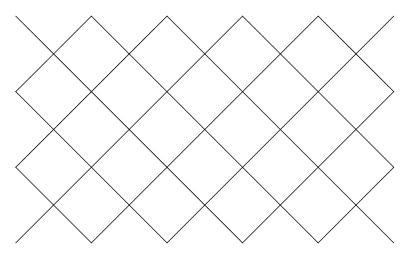
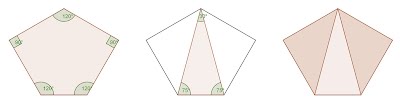
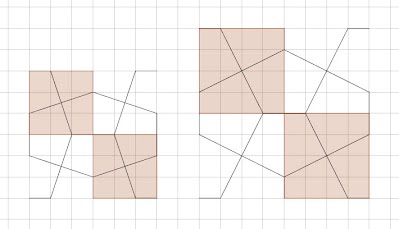
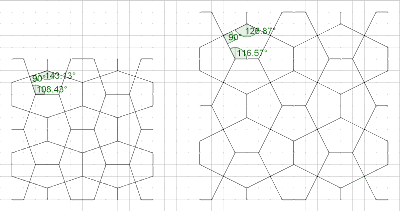
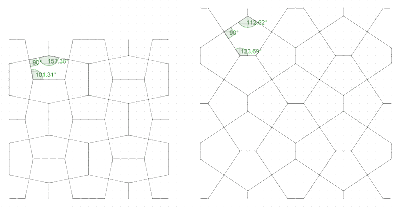
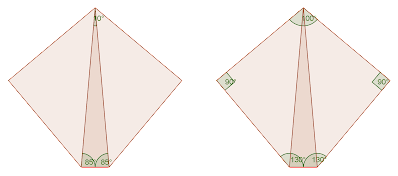
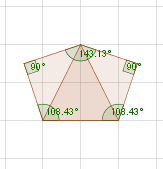
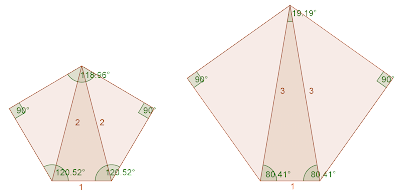
UNDER CONSTRUCTION Of interest is that the in situ model can be described as just one of a family of ‘Cairo-like’ tilings, as the pentagon can vary, or degenerate, with the same broad conditions, from a square to a rectangle (the latter as a basketweave configuration), hence minima and maxima. This feature was first explicitly outlined by Robert Macmillan in his five ‘special cases’, as well as implied (and illustrated) by Herbert C. Moore in his 1909 patent ‘Tile’. However, Macmillan’s account was not of an ‘all-encompassing’ list as such (as I attempt to show below), but rather of establishing the principle, and using instances of ‘special interest’ along the way, namely of the in situ model (with properties of collinearity), an equilateral pentagon, and dual of the semiregular 3.3.4.3.4 tiling. These can indeed be considered as special cases. However, are there more? The term 'special cases’ is open to interpretation. Arguably, although the above can be described as of ‘core value’, there would appear to be others that could be included in this listing. A case in point is the ‘Cordovan pentagon’ with angles of 112.5°, 90°, 135°, 90°, 112.5°, based on the ‘Cordovan proportion’, as (seemingly) first described by the Spanish architect Raphael De la Hoz Arderius, and popularized by Encarción Reyes Iglesias. An open question is to whether there are others (and of which I believe there are) worthy of inclusion. Indeed, I believe I have found another, which I title as the 'Bailey Pentagon'. That said, Macmillan’s list is indeed of the main core values. To this end, I have examined, in different procedures, instances from minima to maxima. This is what I term as ‘incremental angles’ and ‘interstices’. To clarify, ‘incremental angles’, gives a sequence, in ½° increments, whilst ‘interstices’, gives a drawing on unit square graph paper. Both processes find different pentagons; there is no overlap. However, none of these admits the equilateral, of which this is composed by mathematical reasoning. Further, the in situ model is only found by the interstices process. To this end, I present my computations in a series of four main methods in four presentations: angle increments of a rotated cross, square interstices, isosceles triangles and strict mathematical reasoning of a unique case, followed by a table containing all the data. As can be seen, for any one classification, numerous sets of tiles are generated, far too many to be practical to draw all. This being so, I thus show only the more interesting, along with on occasion representative samples, to show extremes. I might just add that this study is typical of many of mine. What begins as a clear and simple idea, (as it undoubtedly is) develops and morphs into something else! Beginning with a minima to maxima premise, this seemingly of necessity morphed into ways of generating Cairo tiles. Arguably, this should be reflected in the title, but for now at least I will retain the original. And then the ‘dreaded drift’ occurs, a not uncommon feature of my studies. In short, the study begins to overwhelm me, and no matter what I do I can make little progress, or even worse, ‘treading water’, where progress effectively grinds to a halt, leaving the study incomplete. Indeed, there are elements to all this here, but here it is. There is always another day, where after a period away from the study I can return refreshed, and more easily see room for improvement. That said, even in its present state, however it may be judged, of its kind of study there is nothing quite like it, by far. Minima to maxima, in all its vagaries, has been effectively ignored. This is at least a considered beginning on the premise. With such a listing, one can examine for patterns, both local, for any one table, and globally, with data for all four. For instance, it is immediately obvious that the Cordovan pentagon is midway (Table 1), at No. 46, and can be considered as the most 'average'. Another observation is the dual of the 3.3.4.3.4 tiling is at No. 61 (Table 1). Therefore, an open question is to the possibility in that is there anything ‘special’ about No. 31, on the other side of the 'dividing' line, separated by the same amount of distance, that has so far escaped everyone's attention? I believe there is, possibly by coincidence, though, and of which I have taken the liberty of making a land grab and calling it the Bailey pentagon! Concerning the diagrams, these will be seen to be at a variety of scales, and typically lack consistency in size. This is simply down to my own shortcomings in drawing in Geometry software, and of which is a necessity for an in-depth analysis here. The only program I can broadly use to some degree of profit is GeoGebra, but for unclear reasons it is a nightmare for consistency between the program itself when exporting as a PNG, resizing in Photoshop, and then finally uploading to Google Sites. Despite what appears to be diagrams of the ‘same size’, in practice this is not found to be so. Many times it is a situation of hoping for the best! Therefore, do bear this in mind with what are at times disjoint diagrams. I have tried my best. That said, the diagrams are indeed correct, but it's just the presentation that is lacking. Method 1 Cairo Tilings by ½° Incremental Angles Method 2 Cairo Tilings formed by Square Grid Interstices by Unit Square Increase. Method 3 Isosceles Triangle - Three Variations: (a) Cairo Tilings by Isosceles Triangles, ½° Incremental Angles, (b) Cairo Tiling by Isosceles Triangles On Square Intersections, (c) Cairo Tiling by Integer Isosceles Triangles Method 4 Cairo Tiling by Squares and Isosceles Triangles. Variations: (a) ½° Incremental Angles, (b) On Square Intersections Method 5 Cairo Tiling of equal sides by ‘Mathematical Reasoning’ Method 6 Cairo Tilings by Projection - Two Variations: (a) Dodecahedron and (b) Spherical tetrahedron Method 1 Cairo Tilings by ½° Incremental Angles Premise - simple incremental angle increase   Fig. 1. e.g. Pentagons of 112.5°, 90°, 135°, 90°, 112.5° and 120°, 90°, 120°, 90°, 120° * LB, SS = Long Base, Short Sides; SB, LS = Short Base, Long Sides ¹ Possesses features of collinearity, see the 'Collinearity' page. ² Possesses features of collinearity, see the 'Collinearity' page. ³ The dual of the semi-regular 3.3.4.3.4 tiling. 91 cases are given, of which most are not particularly of any 'special interest', being a generic Cairo tiling with standard features of overlapping pentagons in hexagons at right angles to each other. However, that said, a few are indeed of special interest, and of which I illustrate and discuss below. Specifically, in sequence: (i) No. 1, where the tiling degenerates to a rectangles in a double basketweave arrangement. (ii) No. 31, a collinearity feature. (iii) No. 46, another collinearity feature. (iv) No. 61, the dual of the semi-regular 3.3.4.3.4 tiling. (v) No. 91 where the tiling degenerates to a square. Interestingly, Robert H. Macmillan, also compiled his own special list, but seemingly failed to notice Nos. 31 and 46, both possessing features of collinearity, but undoubtedly secondary to the in situ model, discussed below. Special cases, as outlined in the comments, from minima to maxima   Fig. 1. No. 1, rectangles, in a double basketweave, left, and Fig. 2. No. 31, Bailey Pentagon, right   Fig. 3. No. 46, Cordovan pentagon left, and Fig. 4. No. 61, Dual of 3.3.4.3.4, right  Another way of looking at this ’incremental approach’ is by observing that if an isosceles triangle is formed from this angle premise, as below, this can also be seen to consist of whole angles, and so the converse of this construction is possible i.e. beginning with an angle-based isosceles triangles of integer angles, such as 75°, 30° and 75°, as below, and then simply attach right-angled triangles to the two equal-length sides. This I show systematically below as a study in its own right.  Fig. x. Showing an alternate interpretation of composing. Method 2 Cairo Tilings formed by Square Grid Interstices by Unit Square Increase. Premise - simple reflection of an equal arm stick cross in a square, and then suitably joined up, e.g. in 3 x 3 and 4 x 4 units  Fig. 2. Two Instances of Reflected Stick Cross Method. Note that there are an infinity of possibilities, arising from an ever increasing square unit size. Therefore, I have restricted my interest to the first few, of 3x3 to 12x12, namely Nos. 1-21. This more than admirably serves to show the main possibilities, prominently of which features the in situ model, No. 1, which is the first instance naturally formed. For convenience, below I show two tables; Table (a) as according to the design sequence, Bailey Nos 1-21, and Table (b) with the data arranged as by the angles. Table 2(a) Cairo Tilings Formed by Square Grid Interstices, Arranged by Unit Square Increase Table 2(b) Cairo Tilings Formed by Square Grid Interstices by Unit Square Increase, Arranged by Angles * LB, SS = Long Base, Short Sides; SB, LS = Short Base, Long Sides Special Cases As above, I now examine these for special cases. However, only one instance can be described as of special interest, namely No. 1 (Table 1a), with a feature of collinearity, and notably this is the in situ model. Below I show four instances, of the beginning, with Nos. 1-2, (Fig. 6) and instances where the base to side length is long and short, as exemplars (Fig. 7).  Fig. 6. Bailey Nos. 1 and 2  Method 3 Cairo Tilings formed by 'attaching' right-angled triangles to an isosceles triangle Premise  Fig. x. Of a core isosceles triangle (here with sides of 1 and 2), two right-angled triangles are suitably attached. No matter if the isosceles triangle is of integers, as here, or of arbitrary sides, or composed by angles, a tiling Cairo tiling is always formed. The above procedure thus gives three variations, as shown below Three Variations: Cairo Tilings by (a) ½° Incremental Angles, (b) integer sides (c) arbitrary Isosceles Triangles, (d) on Square grid Intersections (a) ½° Incremental Angles As can be seen, these are the converse of the 'whole pentagon', as above. Some Examples   Method 3 Isosceles Triangle - Three Variations: (b) Cairo Tiling by Isosceles Triangles on Square Intersections Another possible way of generating distinct Cairo-like tiles is the converse of the above, in that it can be observed that one interpretation is that the pentagon can be divided into an isosceles triangle and what can be interpreted as two right angled triangles ‘attached’ to the sides. Based on this premise I now examine this possibility (Fig. 8). However, it can be seen that these repeat as above, and so I thus show only a single tile instance.  Method 3 Isosceles Triangle - Three Variations: (c) Cairo Tiling by Integer Isosceles Triangles Another possible way of generating distinct Cairo-like tiles is by using an integer isosceles triangle. However, there is nothing in the way of any special features and so I only show the first few instances, of a short base and long sides. Of course, one can also have a long base and short sides, but the same outcome ensues. 
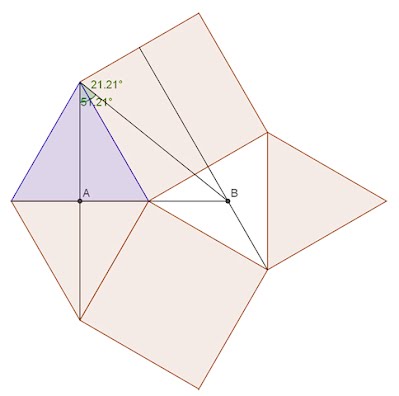
Method 4 Cairo Tiling formed by Squares and Isosceles Triangles. Another way of generating Cairo tiles is by observing that the 120° instance can be derived from the dual of the semiregular 3.3.4.3.4 tiling, consisting of squares and equilateral triangles. _height-400-width-400.jpg)  Fig. x (a) 3.3.4.3.4 Semiregular Tiling, (b) Dual of the 3.3.4.3.4 Semiregular Tiling  Fig. x Minimal block of squares and equilateral triangles with the 120°, 90°, 120°, 90° 120° Cairo tiling To this end, the equilateral triangle can be regarded as an isosceles triangle (although there appears to be some debate), and so as can readily be seen that squares and (generic) isosceles triangles tile together as a tiling, I now investigate this aspect. As according to square grid intersections, integer isosceles triangles, and incremental angles.
Some instances  Fig. xxx. Integer Sides: 1,2, and 1,3   Here I show the minima and maxima by which the equilateral triangle, in blue, can vary. As can be seen, with points A (minima) and B (maxima), it has a relatively limited range. As the angles formed are not rational, this does not lead to an easy drawing of successive increments, and this being so, and with little likelihood of anything 'interesting' arising from this, I thus do not show any resultant tilings. Method 5 Cairo Tiling by Mathematical Reasoning In contrast to the above, where for any one condition there are a multiplicity of tilings, it is possible by analysis to derive an equal sided pentagon, as outlined by McMahon and Macmillan. Macmillan gives a rather advanced explanation, way beyond me, although it is indeed possible to construct easily, as shown by MacMahon. The pentagon is unique.  Table of 'All' For convenience, I here show a table of 'all', or at least the most interesting instances, namely that of (91) ½° incremental Angles of a rotated cross, (21) square Interstices, (91) ½° incremental isosceles triangles, and (1) equilateral. This now permits an assessment to be made. Only a relatively few are of interest, namely the in situ model, equilateral pentagon and dual of the 3.3.4.3.4 tiling. These can indeed be considered as special cases. However, are there more? The term 'special cases’ is open to interpretation. Arguably, although the above can be described as of ‘core value’, there would appear to be others that could be included in this listing. A case in point is the ‘Cordovan pentagon’ with angles of 112.5°, 90°, 135°, 90°, 112.5°, based on the ‘Cordovan proportion’, and my own claim to fame, the Bailey pentagon. Both these have lines of intersection landing on a vertex of a nearby pentagon. Admittedly, both lack the elegance of the in situ model, but nonetheless remain of interest. Stating an overall best is not straightforward. The three main candidates, the in situ, equilateral and the dual, all possess unique attributes that could claim as the best: In Situ - Posseses collinearity of sides Equilateral - Sides of the same length Dual - An elegant construction from the semi regular tiling, although also found by angle analysis Each possesses and excludes attributes of the others. However, if pressed, I would favour the equilateral. Fro me the elegance of equal sides triumphs over the others. * LB, SS = Long Base, Short Sides; SB, LS = Short Base, Long Sides * Arbitrary drawing, to show past core value instances (namely the dual of 3.3.4.3.4 tiling and Cordovan Pentagon), towards minima and maxima, but not all, as all would take too long to draw. * * GeoGebra References Dunn, James. ‘Tessellations with Pentagons’. The Mathematical Gazette, Vol. 55, No. 394 December 1971, pp. 366-369 Gardner, Martin. Scientific American. Mathematical Games, July. ‘On tessellating the plane with convex polygon tiles’, pp. 112-117 (p. 114 and 116 re Cairo aspect), 1975 Macmillan, Robert H. Mathematical Gazette, 1979. ‘Pyramids and Pavements: some thoughts from Cairo’, pp. 251-255. MacMahon, Percy A. New Mathematical Pastimes. Cambridge University Press 1921 and 1930. (Reprinted by Tarquin Books, 2004) Raphael De la Hoz Arderius. 'La proporción cordobesa'. Acta VII Jornadas Andaluzas de Educación Mathemática. Thales, 1996, pp. 67-84 (NOT SEEN) Schattschneider, Doris. Mathematics Magazine, January. ‘Tiling the Plane with Congruent Pentagons’, pp. 29-44 (p. 30 re Cairo aspect). Moore, Herbert C. United States Patents 928,320 and 928,321 of 20 July 1909 Page Created 30 June 2020. Last Updated 2 July 2020. 15-16 July added illustrations |
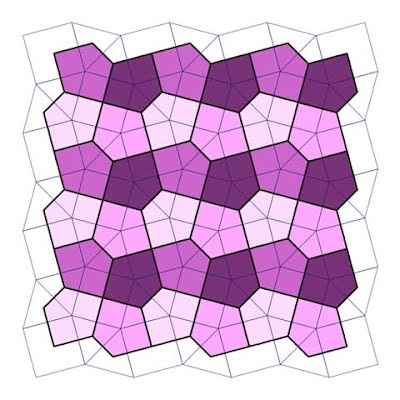
Cairo Tiling >