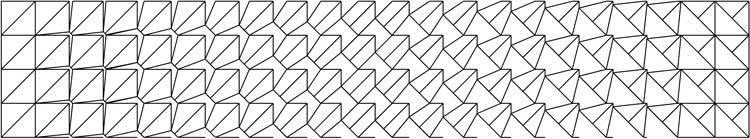
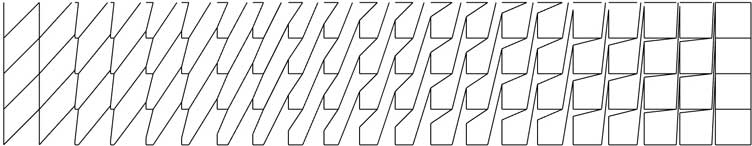
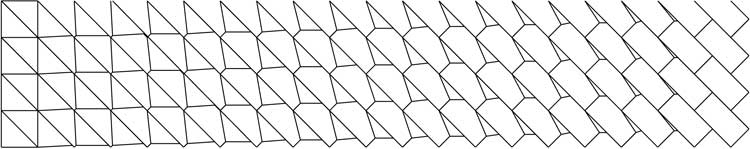
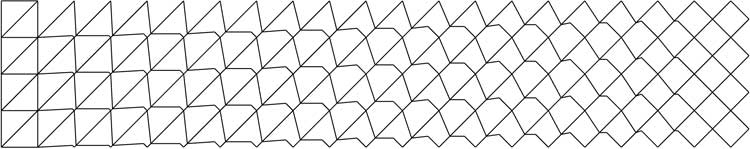
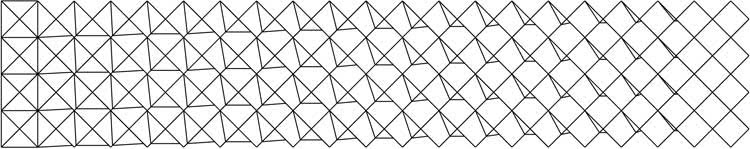
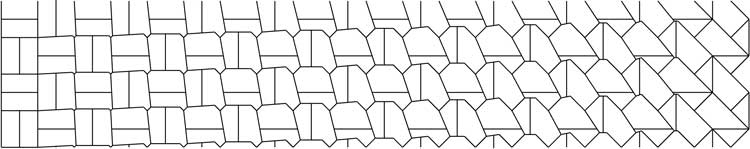
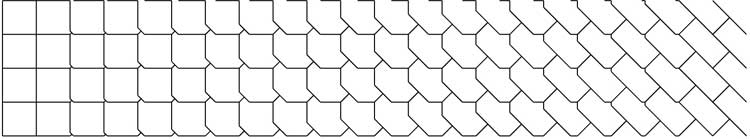
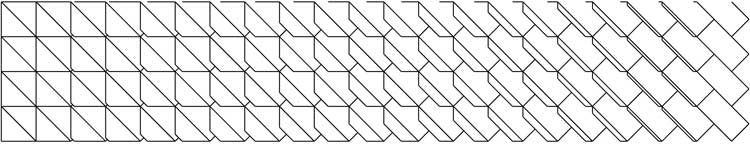
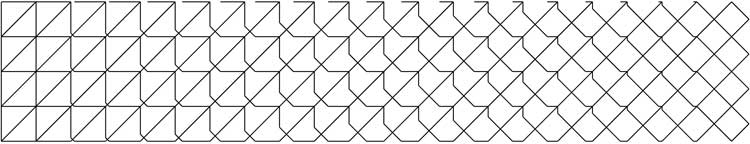
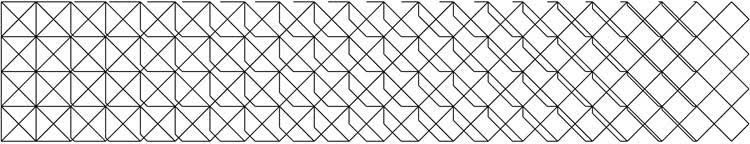
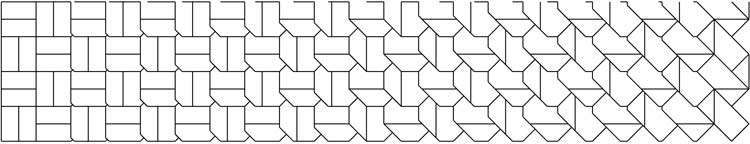
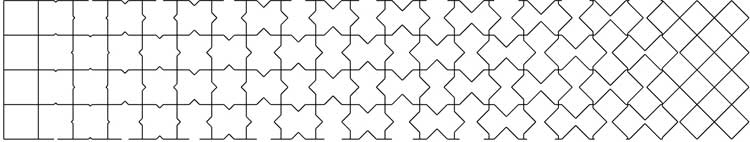
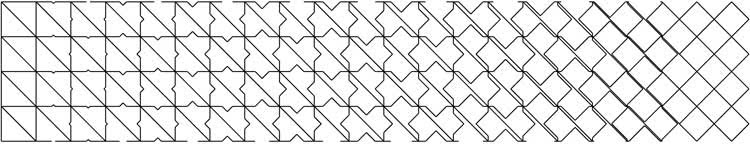
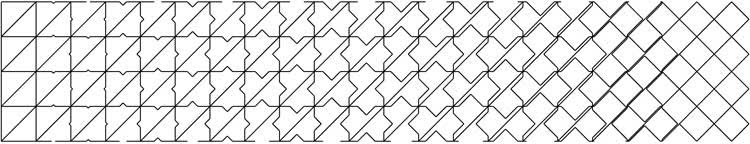
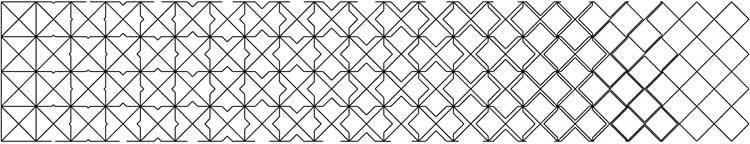
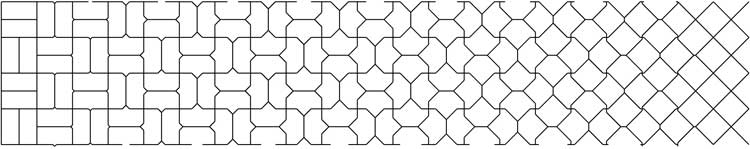
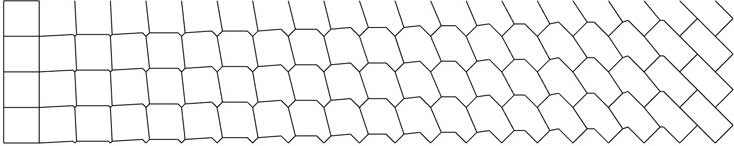
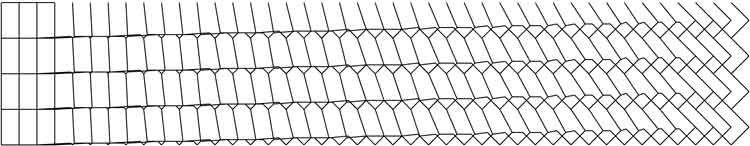
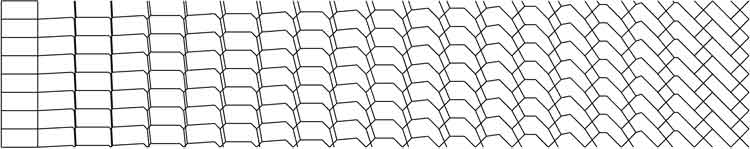
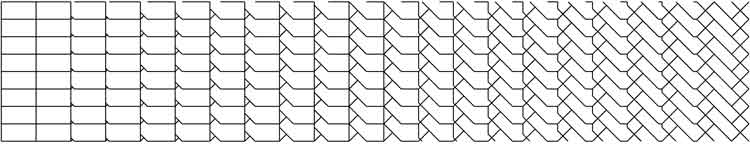
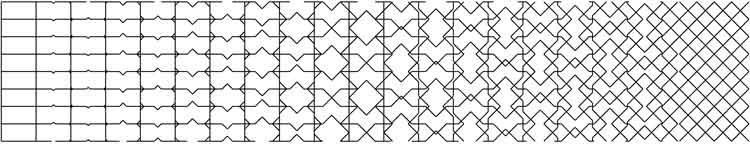
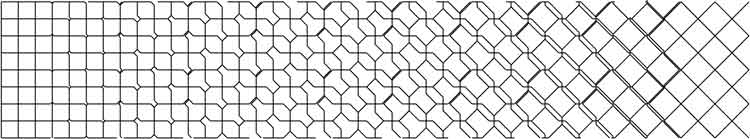
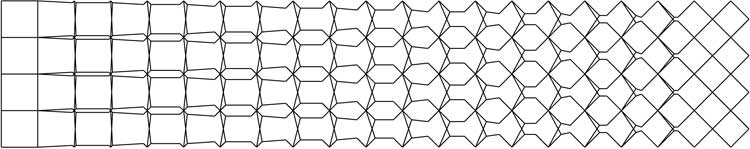
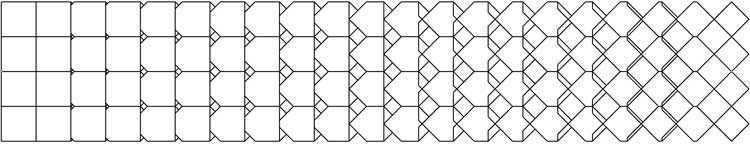
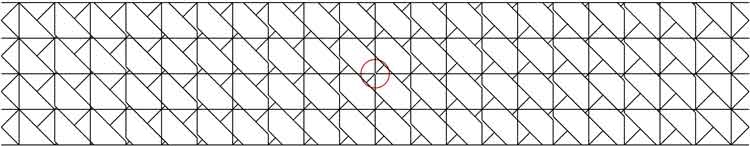
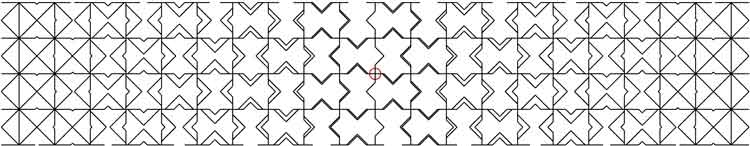
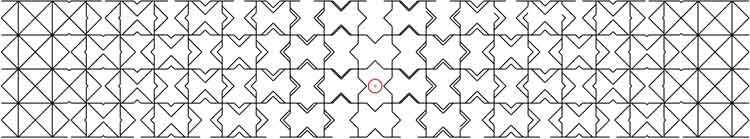
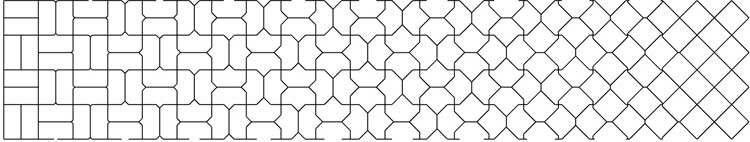
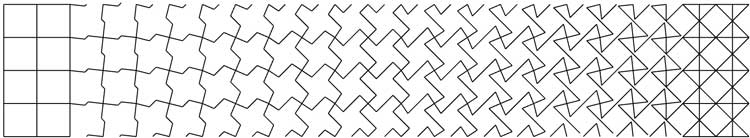
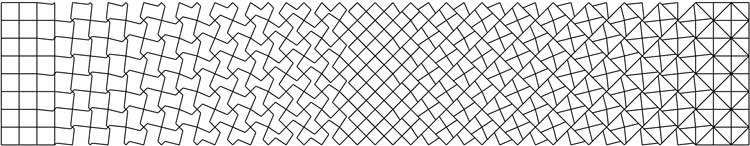
As such, there is very little discussion as to the nuances and intricacies of parquet deformation design, even from William Huff himself. Recently (2021), I have been invited to contribute an 8–10 page article, of a tutorial premise, for a forthcoming book on the subject, by Werner Van Hoeydonck. In the course of this, I compiled a draft containing some aspects of relevance, such as defining a parquet deformation (which is more difficult than may otherwise be thought), the advantages of the computer (as against hand drawn instances), and more. However, not all of this material will be retained in the article. Be that as it may, the text still serves as an excellent guide as to the many nuances and intricacies, and so I have decided to place here under the generic title of ‘Essays’, pending a more expansive, dedicated piece of writing for each topic. Each essay is of a self contained nature, independent of others. To this end, each this has its own set of references. Contents 1. Defining a Parquet Deformation 2. The Advantages of the Computer 3. Experimentation 4. The Two Types of Huff-Inspired Parquet Deformations 5. The Strip Width 6. Colouration 7. Viewing 8. Aesthetics 9. Transition Zones 10. Further Possibilities (Subdivision, Mirror, Rotations, Translation) 1. Defining a Parquet Deformation I begin by first repeating Huff’s definition in Hofstadter [*], which differs a little from my own, but not in fundamentals, and contrasts our definitions. Huff: The deformations are not arbitrary but must satisfy two basic requirements: (1) there must be change only in one dimension, so that it is possible to see a temporal progression in which one tessellation gradually becomes another, and (2) at each stage the pattern must constitute a regular tessellation of the plane, that is, there must be a unit cell that could combine with itself so that it could cover an infinite plane exactly. Bailey: A parquet deformation is one in which a regular tiling of the plane (or more rarely a non-periodic tiling) gets deformed progressively, typically in one dimension as a strip, although two dimensions are permissible. The general process is a tiling as a column, followed by a column as a transition region, of which the process then repeats until the deformation has run its course. The transition column, which contains tiles that possess elements of the preceding and following columns, will thus not tile by itself. I have quibbles with the two points given by Huff. In (1), the main difference is that he rules out two dimensions. Curiously, though, in practice he breaks these rules; there are many two-dimensional instances from his students. In (2), I find this imprecise. As change is involved, there must inevitably be a loosening of the tiling condition. Huff also adds another condition, of ‘allowed parquets’. Upon correspondence, he informed me that some of mine broke his rules [*], [*]. 2. The Advantages of the Computer The computer has many different advantages, depending on mathematical ability, but at its basic is accessible to all. There are * main reasons for this: (1) At its most basic, speed. With copy/paste, much more can be achieved than otherwise, for example, of my favoured 4-unit strip, detailed below, simply drawing a single row, and then repeating three times, obviously only requires a quarter of the time by hand. By hand, the process is most laborious. (2) Productivity. The time saved as above can be used to draw more works, using the example above, four times as much. (3) Error-free work. With undo/redo mistakes can easily be removed that a work with a pen is otherwise impossible, of which with many lines can so easily occur, not to mention the additional fatigue factor. (4) It encourages experimentation that would otherwise be impractical by hand. An instance as such is an assembly into ‘super deformations’ as discussed below. And further, other configurations. Even so, when learning, pen and paper has an immediacy that the computer lacks. Be that as it may, once the basic principles have been grasped, it is relatively easy to transpose those onto the computer. A lot depends on one’s experience. Certainly, CAD programs seem better suited than illustration (e.g. Adobe Illustrator) and geometry programs (e.g. GeoGebra). And further, in the right hands, the computer can be used to expand on the ‘simple premise’ Huff instances. The work of Kaplan [*] exemplifies this, and, to a lesser degree at least in terms of the extent, Edmund Harriss [*]. However, Kaplan’s work, involving advanced algorithms, is somewhat of an outlier. Harriss’s work is on aperiodic tiling. Furthermore, there is the Parakeet plug-in as shown by Esmael Mottaghi and Arman Khalli Beigi [*]. A major advantage here is in the swiftness of execution; a completed, intricate deformation once set up can be composed instantly that otherwise undertaken in Huff’s day would require many hours, if not days! Another computer approach is with a specific goal in mind e.g. deform a given Laves tiling to another. Kaplan [*] shows ten of the eleven possible instances (the eleventh was in ?). However, this typically involves more advanced knowledge required, way beyond most design-focused people. Typically, the first approach will be invariably adopted by the typical design student in the field. 3. Experimentation A feature of the Huff process is that the deformations arose as a result of experimentation i.e. without a goal polygon/s in mind (as against, say, beginning with a square to a rectangle). By this, I mean that once the deform is started, with any of the standard devices, the outcome is not predetermined in a visual sense; only upon completion does it become obvious. Hofstadter himself alludes to this [*]. As a result, not all deformations are as picturesque as another. Some flow smoothly, with elegance, whilst others simply jar. 4. The Two Types of Huff-Inspired Parquet Deformations The Huff-inspired studies can be described broadly as of two types, of ‘simple’ or ‘intricate’. Both types can be seen in Hofstadter's article (some are of a dual placing, as blurring occurs): e.g. Fred Watts’ Fylfot Flipflop and Noel Japach’s Arabesque, respectively. As a rule, I favour drawing the simpler type, in both aesthetics and practical terms. To me, the complicated instances appear to be an exercise in intricacy just for the sake of it. The time taken, for what must have been countless hours, is out of all proportional as to inherent worth. I could easily imagine that this would require the same time as say of ten or more simple deformations. I can certainly admire and appreciate it, but can the time expanded be justified? For me, no. Further, such instances by hand can now be considered as outdated. Esmael Mottaghi and Arman Khalli Beigi show in their Parakeet plug-in (for Rhino) that such types can now be composed, once set, instantaneously. On the grounds of near impracticality by hand and the speed and efficiency of Parakeet, I summarily dismiss such types in the following tutorial. 5. The Strip Width An open question is to the ideal thickness, in terms of units, of the strip. Again, as with tempo matters, this is subjective; there is no one single best. A minimum strip, of 2 units, is judged too brief. Although this is sufficient to show the tiling premise, this is only barely so and can be considered as parsimonious in the extreme. On the other hand, a strip of say 10 units (or more) is judged more so than is necessary to show the tiling premise. In practice, it will be found that 4 units (Fig. *), commensurate with a square here, are judged ideal. However, 6 units are still an eminently viable proposition, and perhaps even 8 units. A tiling rule that I follow, of my own devising, transposed to parquet deformations, is that when one can see that the repeat nature is obvious then there is no need to show more, and so 4 units are judged as ideal. 6. Colouration Invariably, the Huff student-inspired deformations are shown without colour, but this is not discussed in the literature. However, there is a reason for the omission. In private correspondence [*] he told me: Incidentally, while we were studying "pure" parquets---no deformations, we did color many of them black and white, etc. But when we concentrated on the parquet deformation, we did not "color" them, because we saw the beauty in the line.....the shapes of the tiles were secondary...not unimportant, but secondary. One can surmise here that colour would be a distraction from the core premise. Be that as it may, colouration remains a possibility, essentially as an addition, or an option, to the core-value line deformation, but it is not an important matter in itself. Indeed, although the possibility seems obvious, this is generally neglected by the designer. One notable exception is that of Kaplan [*]. Here he shows computer colouration instances, of map colouring contrasting colours, as well as gradients. Another possibility is that of using different coloured lines, where the deformation is in effect an overlay, for example, Fig. * [*]. However, such instances are rare. Again, it should be considered as an addition or option. Add Klee’s quote to this passage somewhere? This reminds me of Paul Klee's famous quote ‘A drawing is simply a line going for a walk’. 7. Viewing Interestingly, Huff wrote on this, linking it with the viewing of Sino-Japanese landscape handscrolls [*]. Typically, with the premise of linear viewing, I favour beginning with the deformation reading from left to right, typically with a square with increasing angularity of lines. Viewing this way, is, to me, more natural, given the western practice of reading left to right. Other cultures read from right to left, and so the deformation should begin at the right. Further, other cultures read vertically, top to bottom, and so in such cases are better presented as accordingly to custom. However, I do not consider the issue a major concern. 8. Aesthetics Of fundamental importance is what I term as the aesthetics of a parquet deformation. In short, whether this has artistic integrity, or, even blunter, is good or bad. It is a relatively easy task (especially with computer assistance) to compose numerous parquet deformations, essentially to the point of triviality. However, not all can be described as aesthetic, which of course should be the standard to aim for. So, what is it that makes for an aesthetic parquet deformation? As such, this is perhaps somewhat subjective; a strict set of rules is difficult to define. Symmetry is a rough guideline but is not infallible. Instances that are symmetrical can be described as aesthetic and non-aesthetic, whilst instances that are non-symmetrical can also be described as aesthetic and non-aesthetic. To aid the discussion, I show instances of the four types, Figs. 1-4. Without too in-depth an analysis, the aesthetic instances, Fig. 1 and Fig. 2, transition from basic, core value tiling polygons of a square to rectangle and two right-angled triangles (set within a square) to rectangles respectively, in a ‘natural’, flowing manner. In contrast, the non-aesthetic instances, Fig. 3 and Fig. 4, although beginning also with core value tiling polygons, two right-angled triangles (set within a square) and a parallelogram, simply do not flow, and can be described as jarring and ‘unnatural’, elongated tiles respectively, and furthermore, transition to essentially nondescript tiling polygons within a square. Detailing exactly the differences is not straightforward, but the most obvious test is a simple, visual one, of which at-a-glance matters of aesthetics are, or should be, self-evident. Fig. 1. Aesthetic, Symmetrical    9. Transition Zones A basic premise of a parquet deformation is that of tiling, and of which Huff specially refers to, in Hofstadter [*]: The deformations are not arbitrary but must satisfy two basic requirements: (1) there must·be change only in one dimension, so that it is possible to see a temporal progression in which one tessellation gradually becomes another, and (2) at each stage the pattern must constitute a regular tessellation of the plane, that is, there must be a unit cell that could combine with itself so that it could cover an infinite plane exactly. However, this is not made clear in visual form. Although Huff’s comments are indeed of the guiding premise, I find his comments and intentions are a little imprecise, and open to interpretation. One interpretation is that all the tiles in the deformation will tile. However, this is not so. By its very nature, with changing tiles, it is simply impossible to compose a parquet deformation in which all tiles will tile. Rather, his explanation would have been served with the use of the words transition zone (or transition stage). This serves as the intermediary stage of the deformation in which the tiles do not tile, whereas on either side, they do. (They are close to tiling, but do not.) I would prefer the following, of which I insert ‘transition zone’ to Huff’s text: The deformations are not arbitrary but must satisfy two basic requirements: (1) there must·be change only in one dimension, so that it is possible to see a temporal progression in which one tessellation gradually becomes another, separated by a transition zone, and (2) at each stage the pattern must constitute a regular tessellation of the plane either side of the transition zone, that is, there must be a unit cell that could combine with itself so that it could cover an infinite plane exactly. A diagram below makes this clearer than any amount of words will do. The red colouring shows the transition zone, in a series of columns.  11. Further Possibilities (Two versions, of 13 and 24 April 2021 New (24 April 2021) Upon the completion of the parquet deformation, which in itself can be considered as serving as a finished work, in effect a first generation parquet deformation, it is then possible to use this as a framework for further possibilities. This involves subdividing, and symmetry operations involving superposing, with translation, reflection (both vertical and horizontal) and rotation. Typically, with all, the parquet deformation becomes more involved, with a broad doubling of lines. In effect, such additional aspects can be described as a second generation parquet deformation forming. The possibilities are just about endless, but the outcome is not aesthetic as another, as happens with first generation parquet deformations. Judgements must be made in the matter, otherwise the results become trivial. The broad aim here is to see any trends/tendencies, both favorable and unfavorable (i.e. aesthetic and non-aesthetic), and then apply the favorable instances to other parquet deformation of a broad like nature. To this end, I use three different parquet deformations as exemplars, with the tiles being asymmetric, and one and two lines of mirror symmetry. The format adopted is two-fold: first, I show the first generation parquet deformation, and second, the various 'extra possibilities' (as outlined above) as a second generation parquet deformation (as such, there can be one or more such second generation instances). Such a format makes clear the 'before and after' aspect at-a-glance. As alluded to above, the computer is a great aid here, and this is where it really comes into its own, with multiple use of a single image. Although each of the examples shown here are practical by hand, much more can be accomplished by computer, in both speed of execution and design. SUBDIVIDING By suitably subdividing the tiles, new parquet deformations can be formed. A variety of lies are used, including diagonals, from left to right and right to left and then both diagonals. A particular favorite is a double basket weave structure. This gives a very pleasing overlay effect at right angles. Other subdivisions are less well defined, selected as according to the parquet deformation. The outcome can depend on the nature of the parquet deformation. For instance, some parquet deformations have asymmetric tiles, of mirror symmetry, or order 4 rotation, all of which impinge on the process as to aesthetics. To this end, I show examples of all three instances, with typical subdivisions. As can be seen, only a few can be described as aesthetic. Asymmetric Tile - Source (Tunisia - Sousse)      Symmetrical Tile, One Axis of Reflection - Source (Argentina - Buenos Aires)  Fig. 2a. First Generation Parquet Deformation (Argentina - Buenos Aires)  Fig. 2b. Second Generation Parquet Deformation. One diagonal subdivided left to right (Argentina - Buenos Aires)  Fig. 2c. Second Generation Parquet Deformation. One diagonal subdivided right to left (Argentina - Buenos Aires)
Fig. 2d. Second Generation Parquet Deformation. Two diagonals subdivided, left to right and right to left (Argentina - Buenos Aires)  Fig. 2e. Second Generation Parquet Deformation. Double Basketweave (Argentina - Buenos Aires) Symmetrical Tile, Two Axes of Reflection - Source (Rio De Janeiro)
Fig. 3a. First Generation Parquet Deformation (Brazil - Rio De Janeiro)   Fig. 3c. Second Generation Parquet Deformation. One diagonal subdivided right to left (Brazil - Rio De Janeiro)   Fig. 3e. Second Generation Parquet Deformation. Basketweave 1 (Brazil - Rio De Janeiro) A beautiful design, with the pleasing feature of overlapping blocks of four tiles at right angles, in the same manner as the famous Cairo tiling.
Fig. 3f. Second Generation Parquet Deformation. Basketweave 2 (Brazil - Rio De Janeiro) Another beautiful design, with the pleasing feature of overlapping blocks of four tiles at right angles, in the same manner as the famous Cairo tiling. TRANSLATION  Fig. 4a. First Generation Parquet Deformation (Tunisia - Sousse)      Fig. 5b. Second Generation Parquet Deformation. Translation half unit, vertically (Argentina - Buenos Aires)  Fig. 5c. Second Generation Parquet Deformation. Translation mid cell (Argentina - Buenos Aires)  Fig. 6a. First Generation Parquet Deformation (Brazil - Rio De Janeiro)  Fig. 6b. Second Generation Parquet Deformation. Translation horizontally, half unit (Brazil - Rio De Janeiro)   Fig. 6d. Second Generation Parquet Deformation. Translation mid cell (Brazil - Rio De Janeiro) N.B. The alignment is (naturally) not exact, masked at this small scale of diagram. MIRROR OVERLAY - HORIZONTAL AXIS Source (Tunisia - Sousse) Fig. 4a. First Generation Parquet Deformation (Tunisia - Sousse)  N.B. Reflected about vertex. The same outcome arises if a midside cell is reflected Argentina - Buenos Aires (Source)  Fig. 4b. Second Generation Parquet Deformation (Argentina - Buenos Aires) N.B. Reflected about vertex. The same outcome arises if a midside cell is reflected Brazil - Rio De Janeiro Fig. 5a. First Generation Parquet Deformation N.B. The same outcome arises if a midside cell is reflected  Fig. 5b. Second Generation Parquet Deformation N.B. The same outcome arises if a midside cell is reflected MIRROR OVERLAY - VERTICAL AXIS Given that this symmetry operation results in the second generation parquet deformation being the same at both ends, this is lacking aesthetically, and is not to be compared to the 'standard model', where the tiles differ at the ends (this negates instances where the same tile arises, but through part of its natural cycle, which is aesthetic) Tunisia ADD Fig. 6b. Second Generation Parquet Deformation N.B. The same outcome arises if a midside cell is reflected
Fig. 7a. First Generation Parquet Deformation   Fig. 8b. Second Generation Parquet Deformation ROTATION OVERLAY - 180 Fig. 9a. First Generation Parquet Deformation (Tunisia - Sousse)    Fig. 9c. Second Generation Parquet Deformation -180 around midcell (Argentina - Buenos Aires)    Old 13 April 2021) Upon the completion of the parquet deformation, which in itself can be considered as serving as a finished work, in effect a first generation parquet deformation, it is then possible to use this as a framework for further possibilities. This involves subdividing, and also superposing, using the three types of symmetry: translation, reflection and rotation. Typically, the parquet deformation becomes more involved, with a broad doubling of lines. In effect, such additional aspects can be described as second, third, and more generation parquet deformation forming. The possibilities are just about endless, but the outcomes are not aesthetic as another. Judgements must be made in the matter, otherwise the results become trivial. As alluded to above, the computer is a great aid here. Although each of the examples shown here are practical by hand, much more can be accomplished by computer, in both speed of execution and design. Subdividing By suitably dividing the tiles, new parquet deformations can be formed. Some are more obvious than others. A particular favorite is a double basket weave structure. This gives a very pleasing overlay effect at right angles. Fig. 1a shows a first generation parquet deformation, whilst Fig. 1b shows the second generation subdivision. Fig. 1a. First Generation Parquet Deformation  2. Translation - Superposition Superposition is another effect with pleasing visual properties. A variety of designs are possible, with a variety of different effects, depending upon the selected parquet deformation. Typical translations include vertical or horizontal slides of a half or one unit. Other possibilities include midpoints of the sides, as well as the midpoint of the cell. Fig. 2a shows a first generation parquet deformation, whilst Fig. 2b shows the second generation midcell translation.  Fig. 2a. First Generation Parquet Deformation  Reflection Rotation - Superimposed 1. Computer vs Handrawn Parquet Deformations Since William Huff’s day, with archived hand-drawn parquet deformations dated 1963-1998, the computer has been used more and more in all manner of artistic endeavours. An obvious question to ask is whether the computer can improve, loosely defined, on the hand-drawn parquet deformations and perhaps take this even further, in new directions, and of which I now address. I now have experience of both procedures, although only latterly of computer drawing, of just the past few months, as against 34 years (at intervals) by hand. Even so, I now consider that I have sufficient knowledge to make a conscious choice as to advantages and disadvantages. To begin, the de facto start is a drawing on graph paper, using the five types of lattice (as outlined by Huff [*]), namely a square, parallelogram, rhombic, rectangular, and hexagonal. Typically, a square is used. Huff outlines the pros and cons of the lattices; some are more suitable than others e.g. squares and parallelograms respectively. Sometimes the term ‘grids’ are used, although this appears to be incorrect. The core issue to address is to how such traditional media, graph paper, can be translated to the computer. In short, this is very well, as might be expected for such a basic concept. Further, upon a basic square grid, typically of 2, 10, 20mm graph paper, of 10 units there is little other paper, aside from 1, 5, 10mm paper available. In contrast, the computer has a much more unit grid possible. At its most basic, both procedures use a grid for drawing purposes, both easily available. For computer software, my choice is Rhino 3D, a CAD program. Although this is arguably overkill, with the 3D features essentially of no application, it is the one program I have gotten along with. But likely a lot depends on one’s experience; I can easily imagine illustration programs e.g. Adobe Illustrator and geometry programs e.g. GeoGebra being used as well. However, CAD programs seem inherently better suited to the purpose. Whatever, this is just my personal viewpoint. In short, I consider the computer a near indispensable tool. Although the instances I show below are all feasible by hand, the advantage of the computer is immense, in a variety of ways, of both in the comparable drawing on (typically) squared paper, and perhaps even more so in the subsequent manipulations. There are 5 main reasons for using the computer: (1) At its most basic, repetition. With copy/paste, much more can be achieved than otherwise. For example, of my favoured 4-unit strip, detailed below, simply drawing a single row, and then repeating three times, obviously only requires a quarter of the time as by hand. By hand, the process is most laborious. This largely removes the tedium factor of drawing the same unit again and again. (2) More productive, with time saved. The time saved as above can be used to draw more works, using the example above, four times as much. (3) Error-free work. With undo/redo, mistakes can easily be removed that a work with pen is otherwise impossible, of which with many lines can so easily occur, not to mention the additional fatigue factor. (4) It encourages experimentation that would otherwise be largely impractical by hand, with ‘superimposition’ in a variety of ways, discussed below. (5) It permits assembly into ‘super deformations’, discussed below. And further, other configurations, such as winding strips. Some softer reasons include an unlimited supply of electronic materials and paper. And further in the right hands, the computer can be used to expand on the Huff student-inspired instances. The advanced work of Kaplan [*] exemplifies this, and, to a lesser degree, at least in terms of the extent, Edmund Harriss [*]. However, Kaplan’s work, involving advanced algorithms, is somewhat of an outlier. Harriss’s work is on aperiodic tiling. Furthermore, there is also dedicated software that can assist, with the Parakeet plug-in, as shown by Esmael Mottaghi and Arman Khalli Beigi [*]. A major advantage here is in the swiftness of execution; a completed, intricate deformation once set up can be composed instantly that otherwise undertaken in Huff’s day would require many hours, if not days! However, this particular use of a computer can be said to be advanced, and likely very few design students will possess the knowledge. An open question is whether such computer-drawn instances can be described as art, of which from the early days of its introduction being an age-old contention. Indeed, one can argue, given the perfection of the computer against the inevitable imperfection by hand that this is not art as such; anyone could have done this. Indeed, one can argue that perfection is soulless, obviously not done by a human, whereas when hand drawn, despite the inevitable imperfection, actually has more charm to it. Certainly, Huff did not seem to regard computer drawing as art. I am ambivalent on this. One can argue that the computer is simply another, better art tool. For instance, no mathematician calculates using a slide rule any more; there are simply more accurate and quicker electronic tools to use. They have served their purpose and are now in the past. A like comparison here can be made with traditional drawing tools, which lack the computer means of copy, paste, undo, redo etc. That said for sure, it is still possible to calculate this way and achieve great things. Indeed, I read that the Apollo astronauts took slide rules to the moon! But not their more recent successors. As above, there are better instruments, and so why cling to, for sentimental reasons, to outdated tools? However, a counter argument is that computer drawings lack the personal touch. Indeed, ever since the days of cave art, signatures have been left, of a hand, saying in effect a unique person made this. Both procedures have their advantages and disadvantages. Artists continue to work, or crossover, to both media. That said, there does seem to be a transition to more modern media. And in relation to parquet deformation, I have to say that I am strongly in the more modern-day camp. To me the advantages of modern media far outweigh any human factor, uniqueness by hand. Page Created 11 March 2021 Essay 1 - Computer vs Handrawn Parquet Deformations 10 April 2021. Added Essays 1-7, with Essay 1 moved down. 11 April 2021. Added Essays 8-9 12 April 2021. Added Essay 'William Huff and Parquet Deformation Music Analogies' 13 April 2021. Added Essay 'Further Possibilities' 30 August 2021. Streamlined the page with the removal of what was Essay 10. 'William Huff and Parquet Deformation Music Analogies'. This text has now been expanded to cover all performing arts (dance, poetry and theatre), with a dedicated subpage. |
Parquet Deformations >
