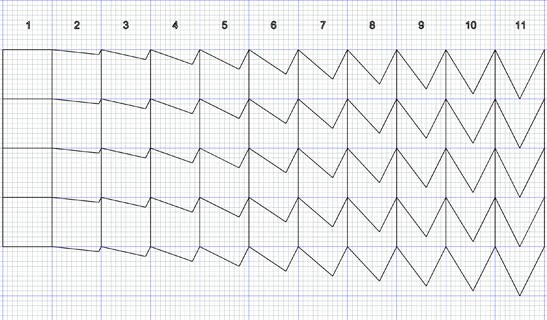
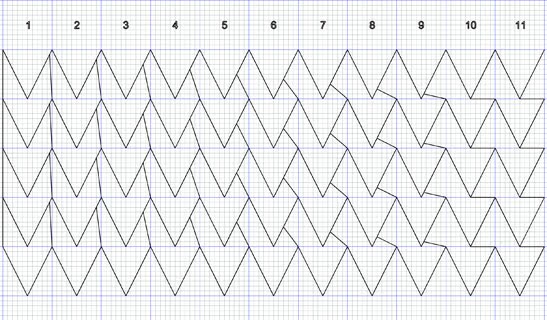
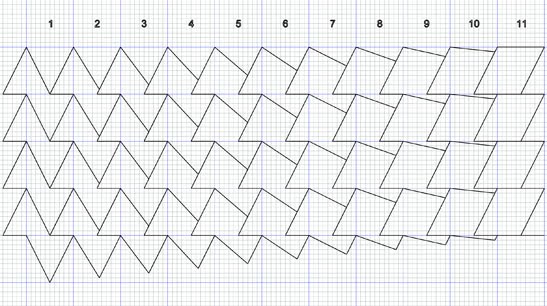
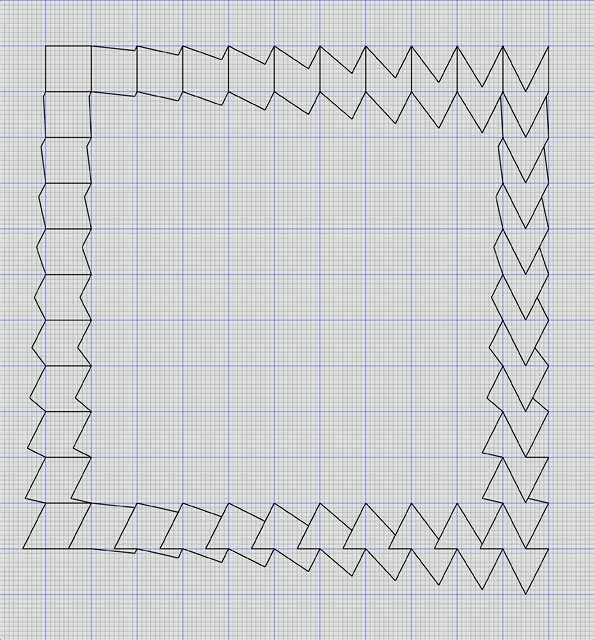
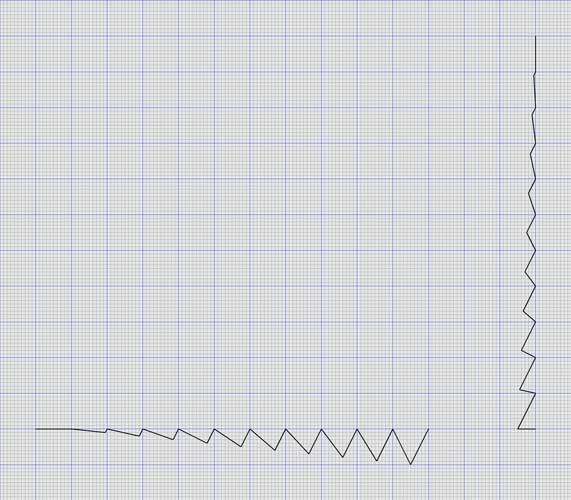
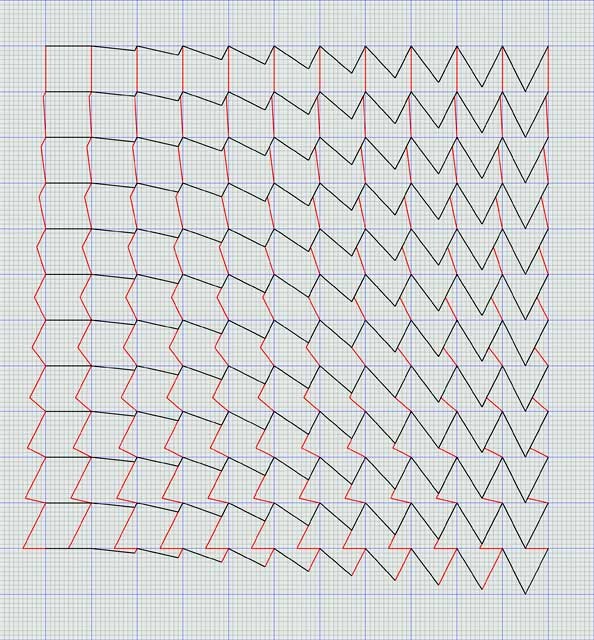
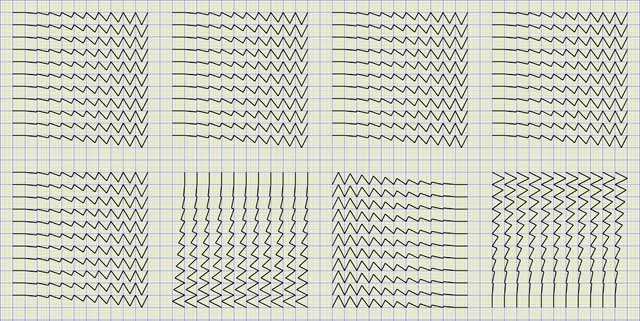
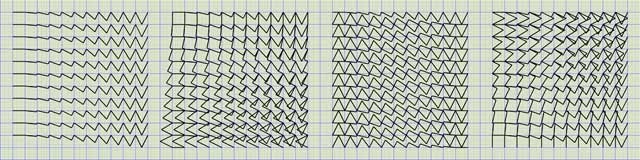
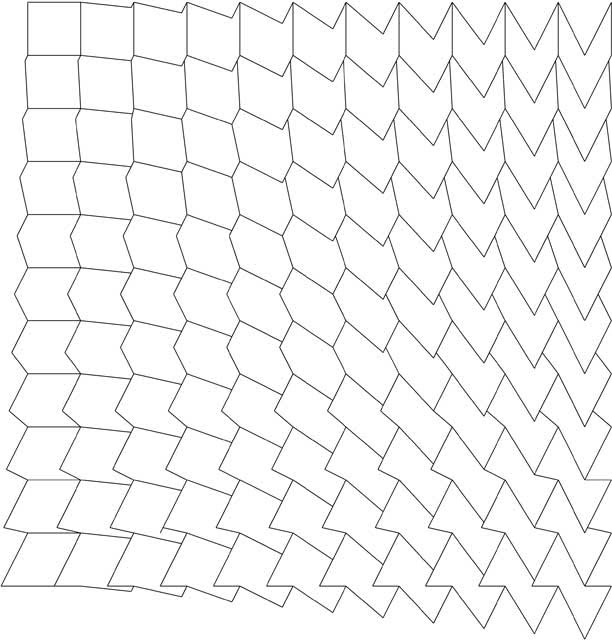
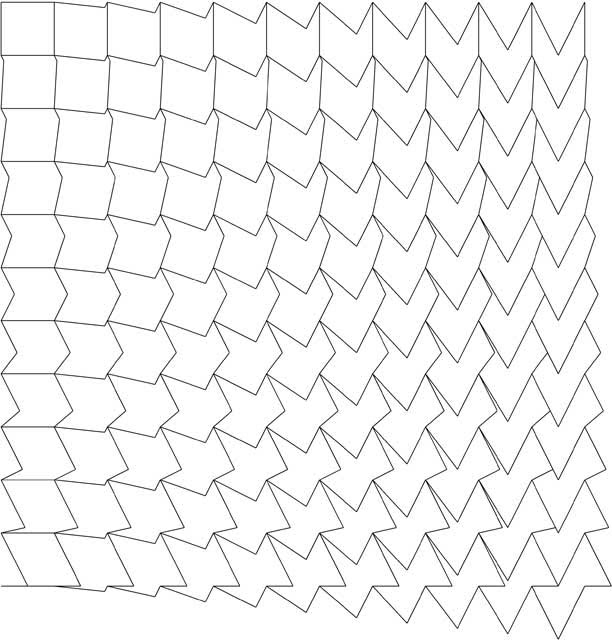
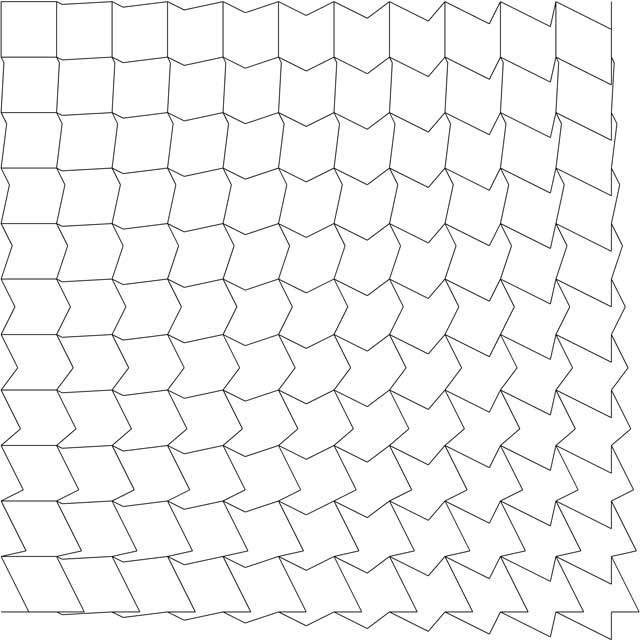
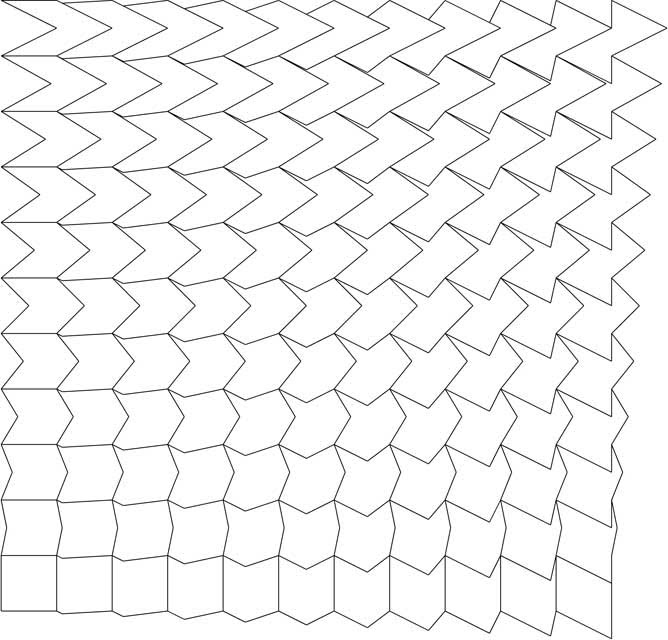
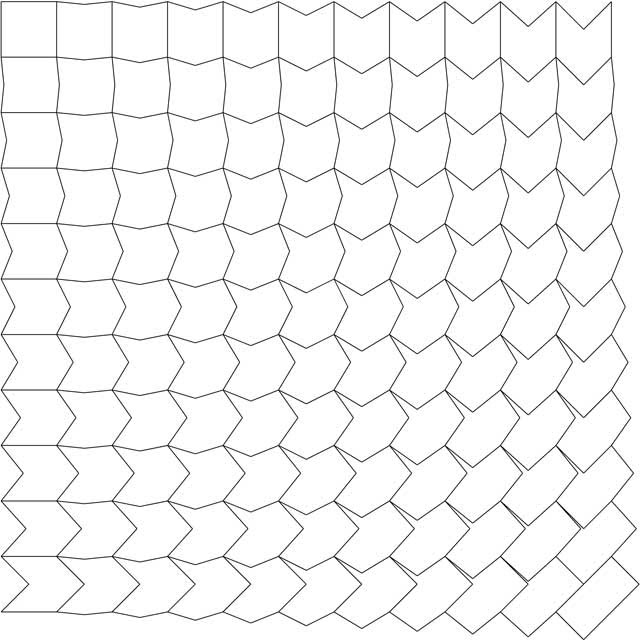
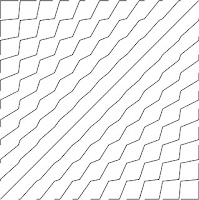
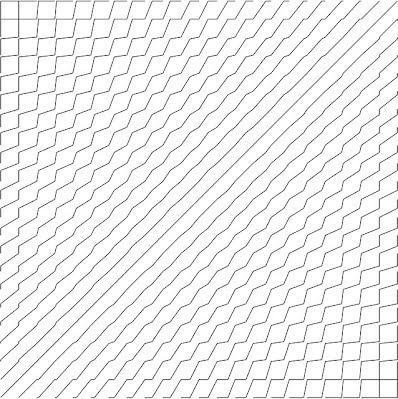
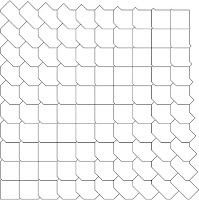
Prelude I’ll begin by stating that this page, as of this June-July 2021 writing (as indeed like most of the sub parquet deformation pages), is in a state of flux (with ongoing studies of various related aspects), and so is subject to review and change. Ideally, I would wait and compose a more settled and polished piece before posting, but this may otherwise take some time, perhaps even many years before this would finally appear, which is perhaps a little too long. As such, I am still forming my ideas on 2-dimensional parquet deformations, and so as well as studying the concept is how best to present my findings. There are considerable vagaries in the concept per se, and so the page will typically evolve, with perhaps material here later placed as distinct studies. And then there are the vagaries of drawing the images, in Rhino, edited in Photoshop, and then onto Google Sites. Preserving the fine grid lines is highly problematic. It does not seem possible to do so without a disproportionate file size, say 1MB. More web-friendly file sizes e.g. 50KB, results in a notable degradation of the image that although (just about) is viewable as webpage, would be unsuitable for the higher standards of an article. As such, I have compromised as best I can. Any suggestions as to improvements/solutions to this problem would be warmly welcomed. Therefore, for the ‘first release’, all the above should be borne with this caveat in mind. However, I have indeed tried to be as thorough and exact as possible in the circumstances. As a broad overview, it suffices but shortcomings nonetheless remain in the finer details in the round. Introduction The next obvious step after the study of 1-dimensional (linear) instance parquet deformations is to move the concept onto 2-dimensions, which I will now discuss. I might just add that a thorough understanding of the principles of 1-dimensions is highly desirable before moving on to this next stage. However, in contrast, such 2-dimensional instances are not perhaps as straightforward in their composing, at least by hand (as contrasting against computer programming, in theory at least; this is beyond my understanding). Indeed, these can be described as of a higher level of difficulty, which perhaps largely explains the relatively few instances seen, as against a widespread (in relative terms) profusion of 1-dimensional instances. Indeed, of the 60 works in the Ulm archive, only 10 are 2-dimensional (listed in the appendix), in a variety of formats, of squares, rectangles, equilateral triangles, and right-angled isosceles triangles. Further, such instances are rare among other people. Of the about 125 people in the parquet deformation field, only Craig Kaplan, Edmund Harriss, Andrew Cooke, Haresh Lalvani and John Sharp have studied the premise but save for Kaplan, only largely fleetingly. Indeed, Kaplan is head and shoulders above everyone here. However, these are typically advanced mathematically, beyond the understanding of design people. As such, there is a very small base point of which to begin the study. Of the ten 2-dimensional works in the Ulm archive, some are more involved than others, indeed so much so that not all of which are conducive to serve as an exemplar, at least of the time I want to devote to unravelling them for the discussion/analysis here. Therefore, following in the footsteps in the analysis of the linear parquet deformations, based on squares, I thus select Leather of the Lesser Gator, by Thomas C. Davies, of 1964 (and incidentally the joint earliest 2-dimensional work) as the most appropriate for analysis purposes. As an aside, the reason for the somewhat obscure title is not clear. Incidentally, there is also another work of a like name Leather of the Greater Gator, by another student, Arthur Carlson, with shared characteristics, also of the same date. From this initial analysis, I then move on to generalities. Reconstruction/Analysis of Leather of the Lesser Gator Leather of the Lesser Gator is arguably the simplest to unravel in the archive, but there are still aspects of it I have not fully unravelled or fully understood. That said, my understanding is more than sufficient to at least give some kind of basic explanation. I begin by showing my reconstruction of the composition showing the underlying grid (Fig. 1) . The actual artwork, seemingly bearing faint, presumed pencil grid lines, of unit squares, and not the finer intersections, is copyright of the Ulm archive, which is why it is not shown here.Of a simple description, beginning top left, of a natural starting point of a square, in a series of four stages around the extremities of the square, the deformation forms a loop, from a square to a chevron to a concave par hexagon to a parallelogram back to the original square. Not only that, the various deformations are consistent in gradation towards the centre, or as an alternative viewpoint, emanating from a central convex par hexagon towards the four extremities. Further, this is based on an 11 x 11 square grid, of ten intersections of each unit. An obvious question to ask is how this was devised. Although Leather is published elsewhere [Hoeydonck], it is not discussed there as such, but rather used as an exemplar of a generic parquet deformation. No explanation is forthcoming from the composer, although this is not exceptional (nor indeed, from any of the artists in the archive). Indeed, so far as I am aware, there has been no discussion worthy of the name in the limited parquet deformation literature (such as it is) on any such 2-dimensional compositions! In my investigations, there are various ways to compose Leather, from, in relative terms, ‘complex’, to ‘simple’, but still within the capabilities of the typical design student. (Somewhat annoyingly, these are not always transposable to other generic 2-dimensional instances.) I will now examine two procedures as to how Leather may have arisen. Method 1 - Assembly of four suitable unit strips As an observation, it is possible to interpret Leather as consisting of four joined parquet deformations of the same tempo (and beat) shown as linear (horizontal) parquet deformations, as below (Figs. 2a-d).Fig. 2(b). Individual parquet deformation of Leather. (b) top right to bottom right. From the individual elements (Figs. 2a-d), I now thus assemble as a 4-unit linear (horizontal) strip (Fig. 3) A possibility, therefore, is that Davis first simply (in relative terms), compiled a four-unit linear parquet deformation as above, and with the observation that the beginning and end tiles are the same, thus formed a ‘square loop’ of a single unit strip (Fig. 4), and then suitably deformed the interior tiles. However, I consider this highly unlikely, albeit still possible. As an observation is the extra amount of work needed to accomplish this in this manner. It is by far from obvious how to proceed from this point. However, there is a much simpler, and quicker, way, detailed in Section 2 below. Method 2 - Modified Zigzag Lines An observation of Leather can also be interpreted in another way, based on just two, slightly different, zigzag lines at right angles to each other!, Fig. 5. The two lines are then repeated (translated) to form a square frame, Fig. 6 (a) horizontally), and (b) vertically Fig. 6. Repeated lines forming a square frame. (a) horizontally), and (b) vertically. Then simply superimpose (a) and (b), Fig. 7, below Fig. 7. Superimposition of horizontal and vertical lines. For clarity, two different colours are used for the two orientations. An (in effect) instant parquet deformation, without the time-consuming procedures of the more long-winded procedure of Method 1! Further, Leather also has an unexpected feature: every tile is a tiler! To me this is counterintuitive; the composition deforms ‘all over’, and yet each tile tiles. This is in contrast to 1-dimension types, where, whether of 1, 2 (or more beats to the bar), all the tiles are not tilers. Specifically, with 1-beat to the bar, no tiles are tilers, whilst 2-beats to the bar tile in alternate columns (the meaning of ‘beats to the bar’ is discussed elsewhere). Therefore, this more complex composition, where arguably even more so a like outcome would also arise, is the opposite to the simpler cases! In hindsight, one can perhaps see why. As the lines repeat both horizontally and vertically, so essentially must the tiles. However, it is a leap in the imagination to recognize this apriori! Certainly, I did not. Indeed, I was tempted to simply dismiss such a possibility out of hand, and simply state that not all tiles tile. After all, some of the interior tiles, with notches, appear unlikely to tile. It can easily be seen that the four corner tiles tile do though, and so perhaps this would be all. Only after a check did I discover that each tile tiles. Below I show four arbitrary examples as 2 x 2 tilings (Figs. 8a-d).  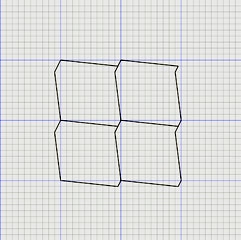  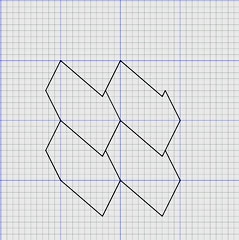 Fig. 8. Four arbitrary tiling from Leather. (a) B7; (b) C2; (c) D3; (d). G7 So How Did Davies Compose Leather?
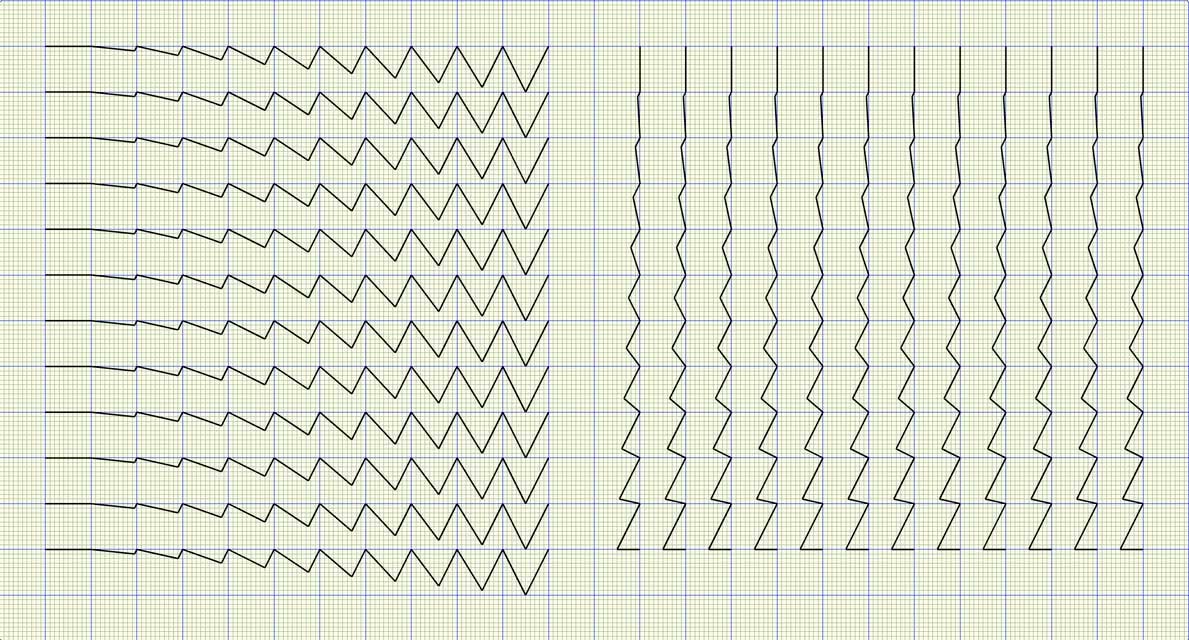
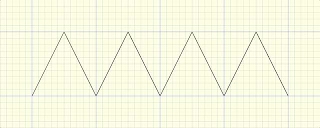
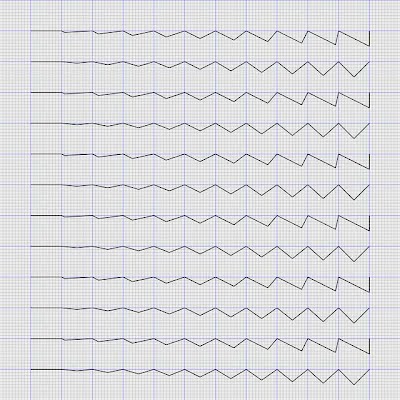
So, to address my original question; how did Davies compose this work? In short, I remain unsure! Cases for and against can be made for both procedures, as above. Intuitively, one would assume the second type, as it is by far the simplest. However, as can be seen, there are many false dawns here; only a few lead to Leather (and the variations). Did Davis just stumble across the Leather lines by chance, of a one-off nature, or did it arise as a systematic study of ‘all possibilities’ in the same vein as myself, as i show below? If so, one might have expected to see more examples, as found by myself, but none are shown. But again, there are some imponderables here. Davies may very well have studied this systematically, but are not shown in the archive. Each student has only one work in the archive. With such scant detail, I am loath to be categorical in attributing any of the above in its composing. For now at least, the question remains open-ended. Progressing and building from Leather Upon the determination of Leather’s likely, or one possible means of construction, an open question is that of building on the premise. Are there other zigzag lines that may be equally as suitable, or perhaps not; perhaps this was just a freak? And just how many distinct zigzag lines are there to examine? Perhaps surprisingly, the zigzag line has received little mathematical treatment. Let us start at first principles; what exactly is a zigzag line? Wikipedia gives A zigzag is a pattern made up of small corners at variable angles, though constant within the zigzag, tracing a path between two parallel lines; it can be described as both jagged and fairly regular. A typical example is shown below, Fig. 9 Fig. 9. Generic zigzag line Symmetry is implied in the definition. However, the lines of Leather do not strictly adhere to the model, notwithstanding the metamorphic element. That said, they nonetheless remain of a zigzag-like nature, and so therefore to have a catch-all description, of these and others of a like nature, I thus define such lines as of a ‘modified zigzag’. Within this framework of a ‘modified zigzag’, an open question is to how many such lines there are. Looking online, there is surprisingly little on the zigzag mathematically. It really is a question of investigating myself. As such, I have identified six lines that are defined as such, Fig. 10. Fig. 10. 6 Distinct modified zigzag lines There may possibly be others, but for now at least I will analyse this as a broad set, with the caveat. Lines 1 and 2 are from Leather. I now show these ‘assembled’ horizontally, as in a square format, Fig. 11. Of course, I could just as easily have chosen a vertical presentation but have chosen horizontal as the default position. From this, it can be seen that to achieve the parquet deformation the lines must be placed at right angles to each other. Now, one could proceed here in superimposing by trial and error, and perhaps by chance stumble upon a permissible composition. However, this is obviously inefficient and unsatisfactory. Although one may indeed find a permissible composition by chance, proceeding in such an hoc manner is unsatisfactory, in that one could not be sure that all permissible possibilities have been discerned. What is needed is a more systematic approach to find all possibilities, and so see if there are more instances of the theme when overlaid, as shown in Fig. 7. This can easily be calculated. Given that there are six possibilities, it is a simple combinatorial problem, as below 1 + 1, 1 + 2, 1 + 3, 1 + 4, 1 + 5, 1 + 6 2 + 2, 2 + 3, 2 + 4, 2 + 5, 2 + 6 3 + 3, 3 + 4, 3 + 5, 3 + 6 4 + 4, 4 + 5, 4 + 6 5 + 5, 5 + 6 6 + 6 And mirror 1 + 1’, 1 + 2’, 1 + 3’, 1 + 4’, 1 + 5’, 1 + 6’ 2 + 2’, 2 + 3’, 2 + 4’, 2 + 5’, 2 + 6’ 3 + 3’, 3 + 4’, 3 + 5’, 3 + 6’ 4 + 4’, 4 + 5’, 4 + 6’ 5 + 5’, 5 + 6’ 6 + 6’ Fig. 12. Top row. Line 1 as a unit block repeated four times. Below row. Line 1 as a unit block in all four orientations. The overlay procedure is simplicity in itself. For each given line I simply superimpose each row of the lines over the trial diagram, Fig. 13. The same process can be used for 1 + 2, 1 + 3, and the other combinations as given. Given that this results in numerous diagrams, I show just a single example here. Typically, these will be found to overlap as here (and so ‘fail’), but on occasion, a 2D parquet deformation in the style of Leather is formed. Fig. 13. Line 1 + Line 1 overlays of top and below rows of Fig. 12. In my investigations, the following combinations below result in ten permissible 2D parquet deformations. However, not all of these are distinct. Nos. 4 and 5 are the same, whilst Nos. 7-10, are effectively so. A listing: 1. Line 1 + Line 2, Position 2 (Leather) 2. Line 1 + Line 2R, Position 4 (This can be described as the inverse of Leather) 3. Line 2 + Line 2R, Position 4 4. Line 2 + Line 5, Position 4* 5. Line 2 + Line 6, Position 4* 6. Line 2 + Line 6R, Position 4 7. Line 6 + Line 6, Position 2 ** 8. Line 6 + Line 6, Position 4 ** 9. Line 6 + Line 6R, Position 2 ** 10. Line 6 + Line 6R, Position 4** * same ** essentially the same For each distinct (or effectively so) instance, I show each in turn below as diagrams, Figs. 12(a)-(e) Fig. 14a. Line 1 + Line 2, Position 2 (Leather). No. 1 of the listing above. Fig. 14b. Line 1 + Line 2R, Position 4 (This can be described as the 'inverse' of Leather, as the same lines have been used). No. 2 of the listing above. Fig. 14c. Line 2 + Line 2R, Position 4. No. 3 of the listing above. Fig. 14d. Line 2 + Line 5, Position 4. Nos. 4 and 5 of the listing above. Fig. 14e. Line 2 + Line 6, Position 4. Nos. 6 of the listing above. Fig. 14f. Line 6 + Line 6R, Position 4 (judged aesthetically the best of the four Line 6 + Line 6 types). Nos. 7-10 of the listing above. Further, echoing Leather, as regards every tile being a tiler, as discussed above, each of these also has every tile as a tiler! Again, the same reasons, in hindsight, must apply; as the lines repeat both horizontally and vertically, so essentially must the tiles. However, note that ‘every tile is a tiler’ is not an invariable outcome of 2-D studies; it seemingly only applies as according to the process of Method 2 (two lines overlaid at right angles). Further Possibilities Further, the general procedure of Method 2, once established, offers many pleasing variations. So far, only one set of lines has been studied for any one square format. An obvious variation is that of two (or more) lines in combination, as in Fig. 13, of Line 1 and Line 2 in alternate strips. However, I have here merely essentially outlined the possibility. Preliminary investigations show a multitude of overlapping lines when overlaid, from which it seems unlikely that these will result in new permissible combinations. Ideally, I would study this in-depth as I have with the single lines, but given that success seems unlikely, with a disproportionate number of diagrams to examine as judged the study is worth, and with the concept a little arbitrary, compared to the core value single lines, I have not pursued this particular study.
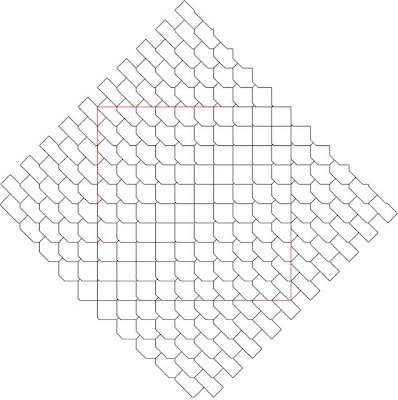
Fig. 15. Line 1 and Line 2 in alternate rows. Another approach to forming 2D parquet deformations - filling in an open grid Yet another approach is that of a ‘modified linear’ parquet deformation. Simply stated, this follows in its (two-stage) linear footsteps, with an open grid and then filling in (Fig. 16(a) and (b). This also echoes that of 1- and 2- Beats to the Bar. However, exactly what I am doing is not easily explained in words. For now at least, I will simply give a largely visual presentation. Fig. 17a. Open Grid - 2-Beats to the Bar Observations Of note here is a large increase in the grid dimensions and as a consequence the number of tiles between the respective 1- and 2-beats to the bar. A quadrupling is in order (and not simple doubling) of the number of tiles, from a grid of 11 x 11 to 22 x 22, and from 121 tiles to 484. Further these echo the nature of the linear 1- and 2-beats, in that 1-beat does not have tiling tiles, whilst 2-beats does (in alternate columns). This feature can also be seen in the 2-dimensions by observing the centre of the square frame, of a rectangle. In Fig. 16b it is not quite a rectangle, whilst Fig. 17b it is indeed a rectangle. Yet another approach to forming 2D parquet deformations - flexing Note that the above procedures are not the only way of designing 2-dimensional parquet deformations. A different way is to essentially retain a single tile and suitably deform it, namely with flexing. An (excellent, and oft-discussed) example is David Oleson’s 1964 work I at the Center. This has been catalogued/discussed/philosophised/recreated by numerous people, first catalogued by William Huff [2], popularised by Douglas Hofstadter [3], and then emanating from Hofstadter’s account, Tuğrul Yazar [6, 7], Premshree Pillai [4], ‘extropiadasilva’ [1], Richard Plender, ed [5], and ‘Varyter’ [8] have all given their thoughts, of which I thus largely defer to here, save for a short statement. Here, the letter I, of a human concept of self, is at the centre of a square format and is progressively deformed, by flexing. A feature here, unlike the variations of Leather, above, is that vestiges of the original tile remain at the extremes of the deformation, at the four corners. Ideally, I would study this myself, but of an initial assessment it appears rather convoluted; I could easily imagine that it may take days or even weeks to unravel, and such time I do not have. Further, the others mentioned above have all seemingly unravelled and recreated it, albeit this is not set out as such. I at the Center, and many others in the Ulm archive, are typical in that although with time most could be unravelled, the time and effort are judged simply too great. It is far easier to undertake original work of a similar type than examining others' designs. Faux 2-Dimensions On occasion, some people make claims as to a 2-dimensional parquet when it is not. Admittedly, at first glance, these instances appear to be so, but upon closer inspection are not. To illustrate the point, here is a typical example I have especially composed for illustrative purposes Fig. 15). At first glance this is indeed 2D, of an apparent ‘all over’ transition. However, this is not so. Rather, this can be considered as ‘excised’ from a 1D parquet deformation (Fig. 17). Fig. 19. Faux 2D outlined in red formed from 1D Parquet Deformation The red square is the outline of Fig. 14. As can be seen, this is a linear parquet deformation, set at 45°, of a transition from rectangles to squares to rectangles. References [1] ‘extropiadasilva’. ‘I at the Centre’. https://extropiadasilva.wordpress.com/2010/08/11/i-at-the-centre/ [2] Huff, William. Ulm archive. David Oleson’s ‘The I at the Center’, HfG Archive Nr. Hu P 06.011 [3] ————. ‘The Parquet Deformations from the Basic Design Studio of William S. Huff at Carnegie-Mellon University Hoschule für Gestaltung and State University of New York at Buffalo from 1960 to 1983’. Unpublished, 1983. ‘Workbook’ (my description). [4] Hofstadter, Douglas R. ‘Parquet Deformations: A Subtle, Intricate Art Form’. July, 1983, pp. 190–199. In Metamagical Themas: Questing for the Essence of Mind and Pattern. Basic Books; First printing 1985, New edition 1996. [5] ————. I Am a Strange Loop. Basic Books, 2007 Hardback, 2008 Paperback, 412 pp. David Oleson’s I at the Center parquet deformation discussion in Chapter 1, ‘How We Live in Each Other’, pp. 241–258. The premise of the book, detailed below, thus makes David Oleson's work here an obvious choice for inclusion. Note that Hofstadter here is making a point in his writing, and is not a discussion as to the parquet deformation in general, as with his Scientific American 1983 piece. [6] Pillai, Premshree. ‘I at the Center’. https://disquiet.in/posts/2019/10/31/i-at-the-center [7] Plender, Richard (ed). Dora Kostakopoulou. Chapter 5. ‘The Capricious Games of Snakes and Ladder: The Nexus of Migration and Integration in Light of Human Rights Norms’, pp. 91-110. See pp. 108-109 (p. 109 Oleson illustration). Issues in International Migration Law Brill - Nijhoff, first edition 2015, p. 108. Preview available on Google Books. [8] Yazar, Tuğrul. 'Revisiting Parquet Deformations from a computational perspective: A novel method for design and analysis'. In International Journal of Architectural Computing. Volume: 15 issue: 4, pp. 250–267, 2017. [9] ————. https://www.designcoding.net/one-at-the-center/ [10] ‘Varyter’. ‘I at the Center’. https://www.reddit.com/r/processing/comments/ajbkah/i_at_the_center_david_oleson_1964_recreated_in/ Appendix. Listing instances of 2-dimensional parquet deformations in the Ulm archive (N.B. not available online) and ‘Workbook’. Only occasionally are instances reproduced elsewhere Sequential 3. Leather of the Greater Gator, by Arthur Carlson. Rectangle, 21 x 10, 1964 4. Leather of the Lessor Gator, by Thomas C. Davies. Square, 11 x 11, 1964 5. Toccata, by Robert Glenn. Rectangle, 17 x 9, 1965 13. One in Threes makes Three or a Star. Equilateral Triangle c. 13 x 13, 1991 14. Crosses Crookedly Crisscrossed. by Jack Holnbeck, ‘Crosses’, 1990 19. All in a Family, by Roland B. Findlay III. Square, 13 x 13, 1965 29. The I at the Center, by David Oleson. Square, 17 x 17, 1964 30. Sitting Ducks, by Lawrence Robinson. Right-Angled Triangle, 16 x 9 x 9, 1978 44. In-tri-cut, by Marsha Berga. Square, but of isometric-based tiles, c. 34 x 34, 1970 50. Stretching a Point or Two, by Thomas Breen. Right-Angled Triangle, 17 x 17, 1993 Only 29 and 50 are in the public domain. 29 can be seen in Hofstadter's Metamagical Themas 50 is at https://www.tom-breen.com/design-research.html Workbook. All untitled, undated and author uncredited. Titles. dates and author given are from works known elsewhere 52. The I at the Center, by David Oleson. Square, 17 x 17, 1964 53. All in a Family, by Roland B. Findlay III. Square, 13 x 13, 1965 54. Anon. Rectangle 16 x 8, Undated 55. Sitting Ducks, by Lawrence Robinson. Right-Angled Triangle, 16 x 9 x 9, 1978 56. Leather of the Lessor Gator, by Thomas C. Davies. Square, 11 x 11, 1964 57. Leather of the Greater Gator (1), by Arthur Carlson. Rectangle, 21 x 10, 1964 58. Leather of the Greater Gator (2), by Arthur Carlson. Rectangle, 21 x 10, 1964 59. Anon. Square 15 x 15, Undated 61. Anon. Triangle (1), Undated 62. Anon. Triangle (2), Undated 63. Anon. Square, but of rhombic, hexagonal tiles, Undated 65. Anon. Square 15 x 15, Undated, ‘Failure’ 66. Anon. Rectangle, but of hexagonal tiles, Undated To be continued. Page created 22 June 2021. Last updated 19 July 2021 |
Parquet Deformations >